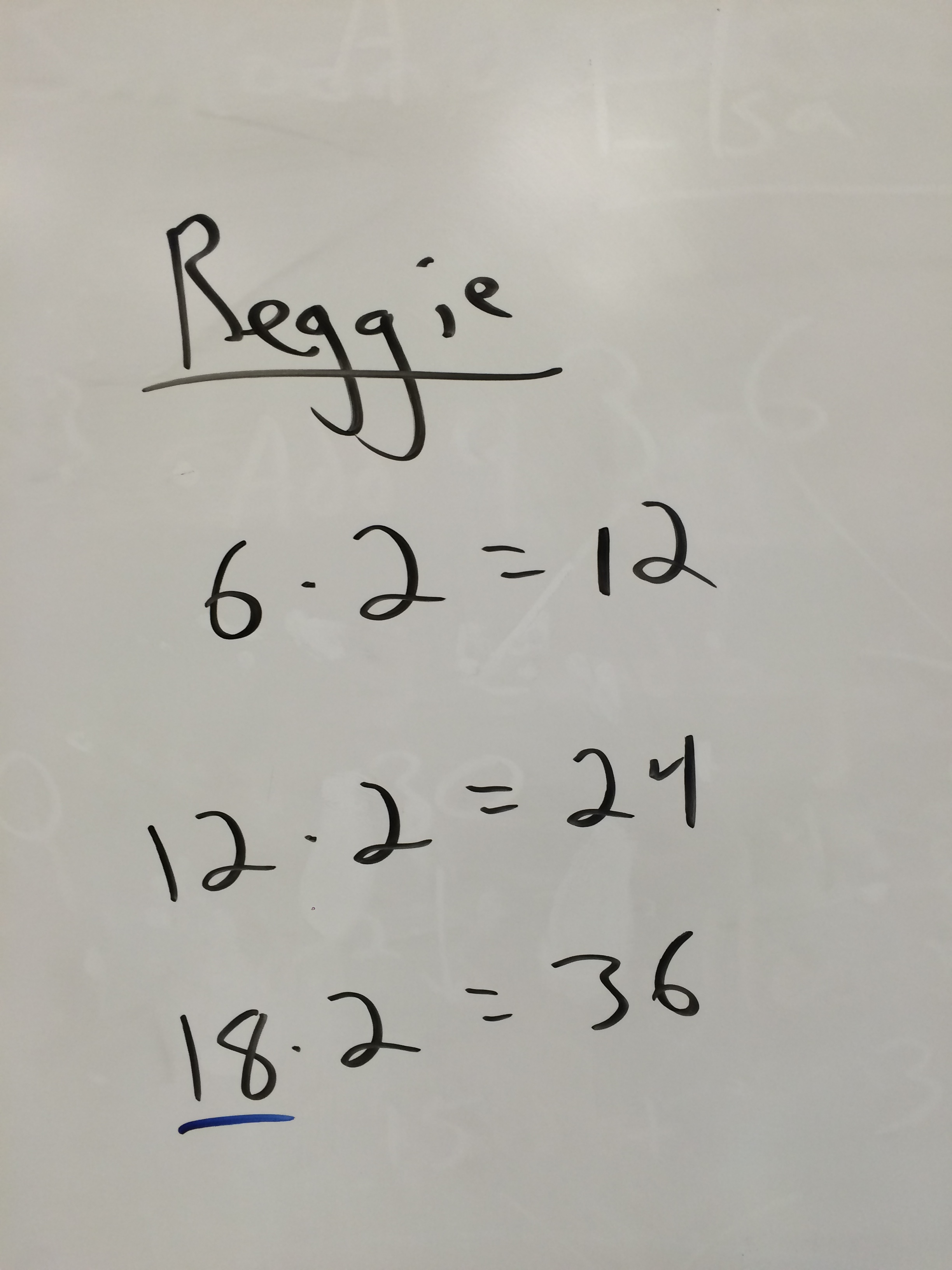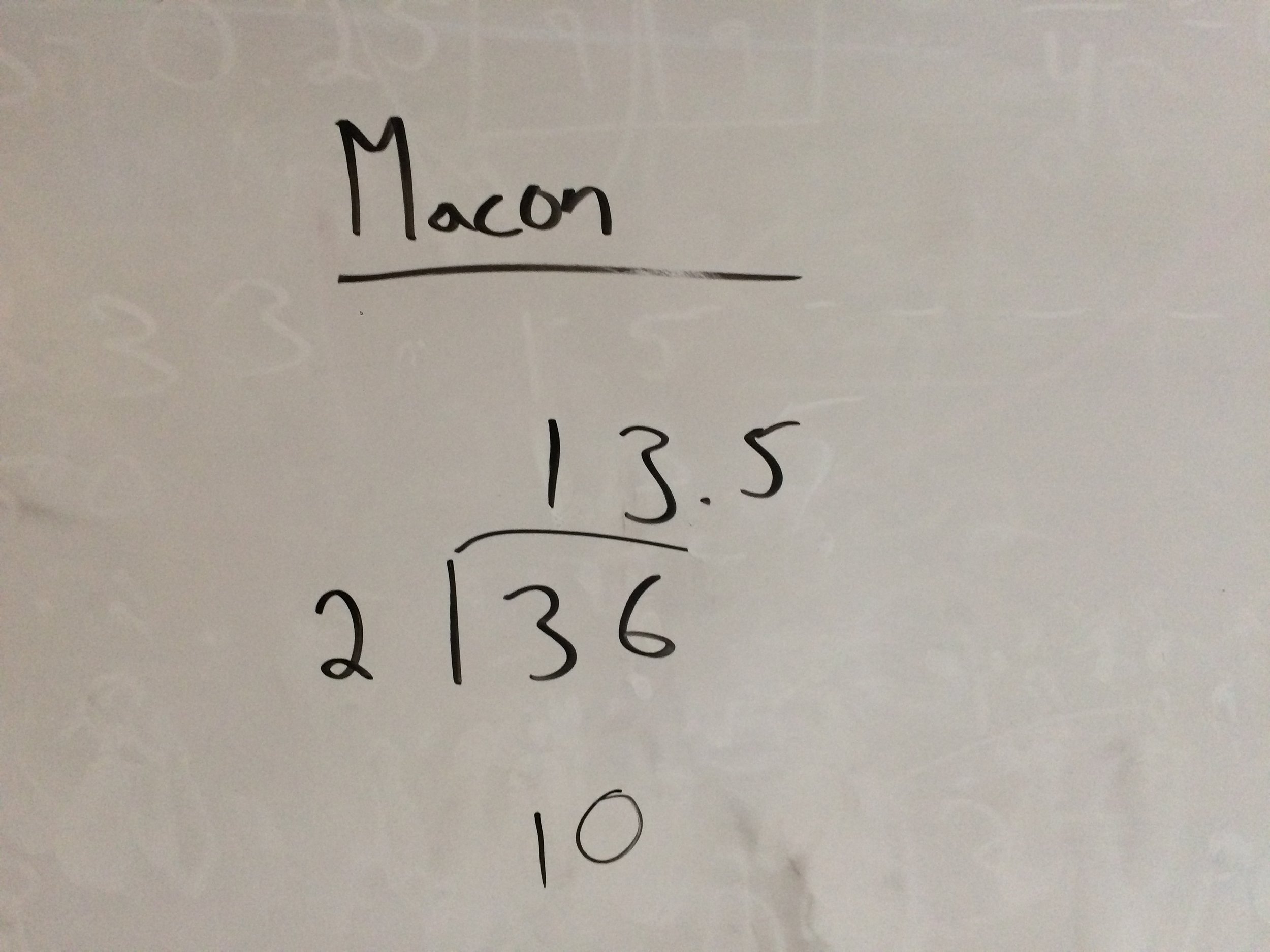Congrats on not using a textbook, Kent. Now what?

In my first job, I taught 6th, 7th, 8th, and Algebra 1 every year. My administration gave me a list of standards for each grade level and a bunch of textbooks, 3 of which were abysmal. (One was pretty good).

So that year, most of the lessons I taught were either compiled from elsewhere or written by me. And for the past seven years in three different schools, it’s pretty much been that way. Aside from that old Algebra 1 class, I’ve never used a single curricular resource as the backbone of my classes. 

Right now I teach 8th grade and Algebra 1. For linear functions, I use Moving Straight Ahead from the Connected Mathematics Project. For the Pythagorean Theorem, I am adapting a unit from a Carnegie book I got a peek at. For expressions and equations, I am adapting a bunch of Heinemann's Transition to Algebra resources (not enough white space). For intro to functions in Algebra, I am combining some Carnegie stuff with Laying the Foundation, a set of curricular resources developed by the National Math and Science Initiative. For various lessons I use Mathalicious or Desmos or Shell Centre or something else. For squares and square roots, I am writing and rewriting my own unit. And I am not the greatest at organization, so I often find myself digging through old binders or Google Drive folders looking for that activity that went so well last year.

It is completely exhausting, and yet I don’t know any other way to teach. I can’t use my district’s current textbooks because they are horrible. We don’t have money for the textbook I would prefer. I asked. So all I can do is write my lessons fresh every night and hope someone listens to me in two years at the next textbook adoption.

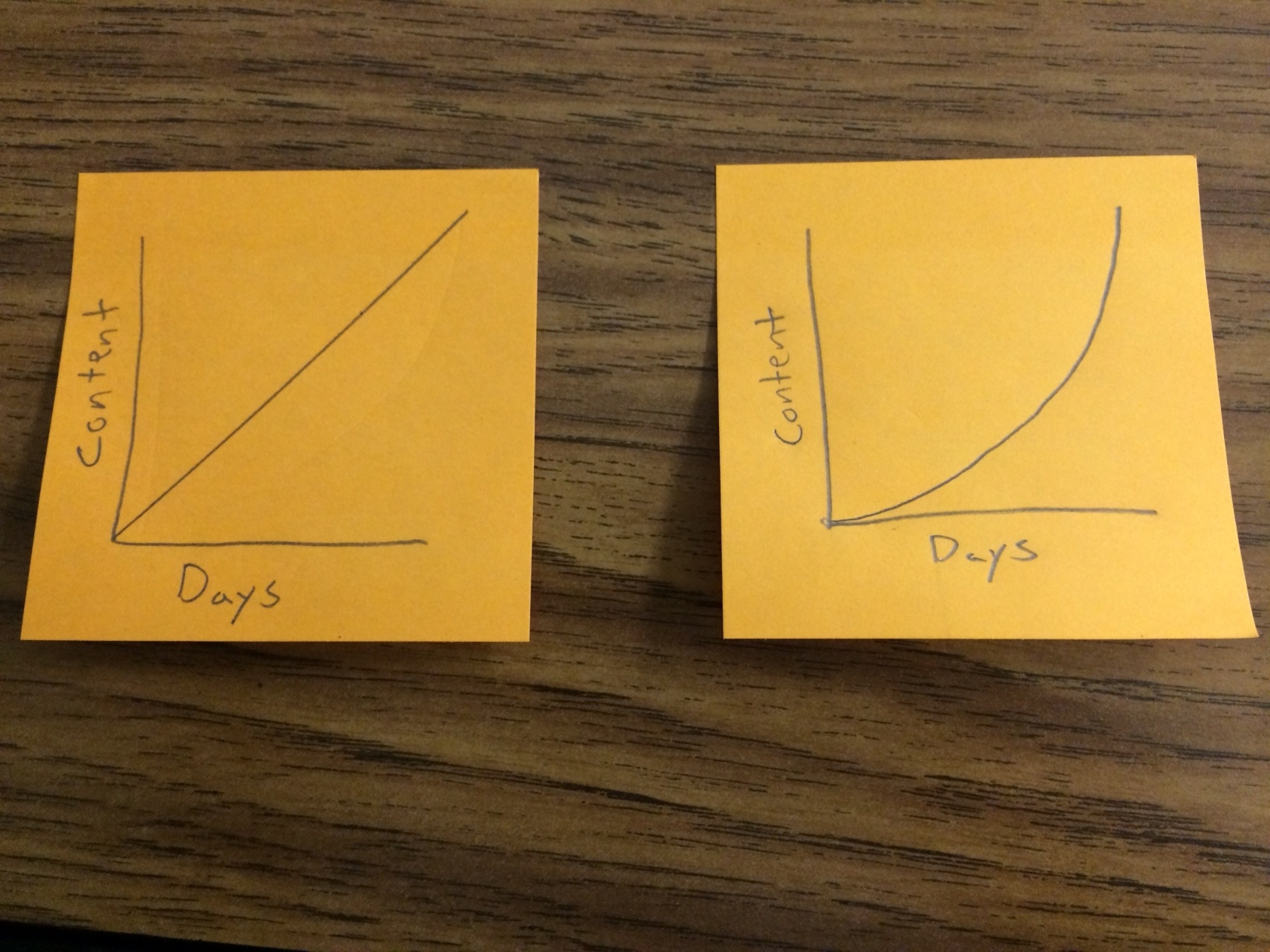
I don’t know how ong I can keep it up. I keep hoping that things will get easier - that I will settle into a groove and be able to edit, rather than rewrite, my lessons. But as I've grown as a teacher, I've started to see that 10 great lessons do not make a great unit. There is a level of coherence that I only just started to really notice, and it doesn't exist in many of my cobbled-together-with-duct-tape units.

I was talking today with a colleague who has been at it for a while now. She has trouble with the textbook and tried to move off it last year, but she sensed that lack of coherence and it really bugged her. So she was telling me that she's back on the book for Algebra 1 this year, but still trying to teach Pre-Algebra with the materials we are all creating or collecting.

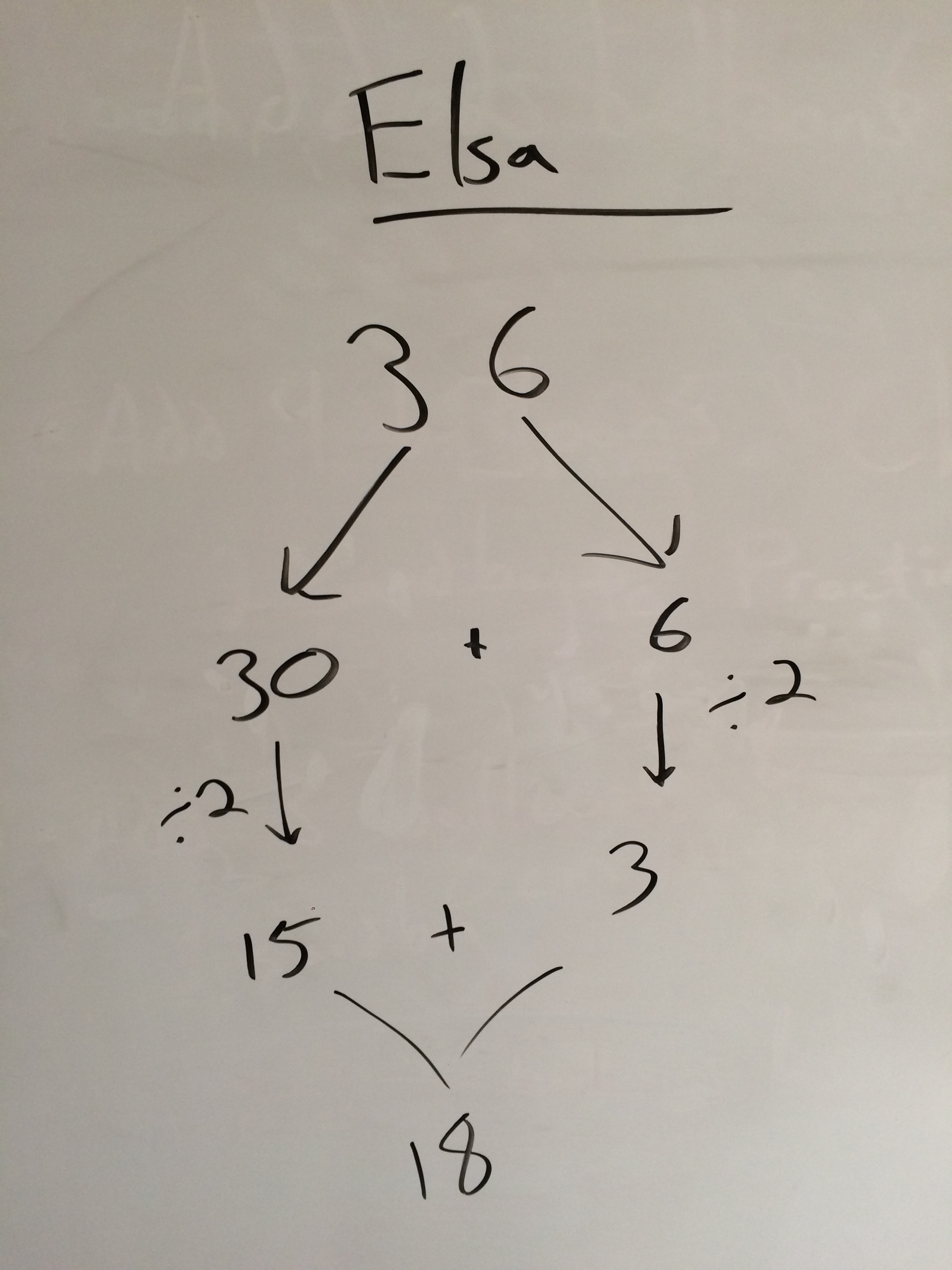
I showed her how I am introducing Unit 2 of Algebra, with some number tricks and mobiles adapted from Transition to Algebra, and she got really excited. "This stuff is great! They will really be able to see why we use inverse operations to solve equations!" She borrowed my worksheet and made copies for her class. 

She reported back this afternoon that the lesson went well - kids were engaged and motivated. She asked if I had any more stuff like that - it's too daunting for her to make whole packets of material like that on her own. Fortunately, I do have more. One day more. Then I'll be at the copier or on Google Docs, cutting and pasting something new for my students and hoping that it all coheres at the end.

My colleague wants to use good resources and teach good lessons. So do I. But I don't know how long either of us can keep it up at this pace. And this sure as hell isn't a model that is going to lead to effective math instruction in every classroom.

I'm not the first teacher to talk about this. Chris has, Dan has, Dylan has, even the President of NCTM has. I don't have an answer. I'm just trying to add my experience to the conversation.

Day in the Life - September 12th

I am contributing to Tina Cardone's Day in the Life series this year. I'll be writing about the 12th of each month.

Today was a little rough.

Some context: My wife spent the weekend at a wedding in Philadelphia, so I was home with my 4 year old son and 1.5 year old daughter. I knew this on Friday and tried to get everything ready for Monday ahead of time, but it felt rushed. So instead of refreshing my memory and refining my plans on Sunday night, I fell asleep 

Then this morning I had to get both kids fed, dressed and out the door by 7am (wife still not home) so that I could get to school by 7:50. My first period came in at 8:05. So needless to say, I wasn't in the groove yet when they arrived.

My Pre-Algebra lesson today is an introduction to the Pythagorean Theorem. In general, I like to start my units slowly. I'd rather make sure everyone is beginning from a place of comfort and then ramp up the intensity towards the end of the unit. This is not how I've always taught, but rather an approach I've developed over time.

Left:How I used to introduce new content. Right: How I introduce new content now.

So I'm not going to use the phrases "Pythagorean Theorem" on day 1. Instead, I hand everyone a slip of paper with this triangle on it.

I ask them to brainstorm everything they know about the triangle in groups. As I walk around I start to hear encouraging words like "perpendicular" and "isosceles." My students, for some reason, are particularly behind in geometry. I don't know if it's a matter of emphasis district-wide or some other reason, but I find that there is no such thing as "too obvious" in 8th grade geometry.

After collecting some ideas on the board, I have something like this:

• Right angle
• Isosceles (two sides the same, one different)
• The short sides are each 5 blocks long
• The area is 12.5 blocks

Now I ask the motivating question for the entire day (and unit, really):

How long is the longest side?

This is the point at which my planning really fell off the rails. I expected my students to notice immediately that the longest side was longer than 5 blocks, but this turned out to be very controversial. You see, the long side does cut through 5 blocks, so some kids thought it was the same length as the others.

I ended up getting a bunch of rulers and asking the students to measure all 3 sides with the centimeter side. We got 6.5, 6.5, and 9 inches as the measurements.

Then one student said "The long side is 7."

Pardon?

"I used my ruler to see how many blocks long 9cm was, and it's 7 blocks. So the short sides are 5 and the long side is 7."

Holy crap - she's right! Or at least, the long side is VERY close to 7. In fact, we are going to prove that very thing today! And she discovered it on her own using a totally different method!

I had lightning in a bottle, but honestly, I had no idea what to do with it. 

Then I looked around the room and saw how my students' engagement level was dipping. So I asked them "Can someone explain E's strategy for finding the length of the long side?"

Nobody could. So I asked E to explain herself again.

I ask again "Can someone explain E's strategy?" Still nothing.

I realize that I have done a bad job of implementing a very important element of classroom culture: the idea that students need to listen to each other.

So I start calling on students at random, asking them whether they can explain E's method. Three students in a row can't explain it, even after E repeats herself to each of those students. Finally the fourth student explains her strategy.

I am running out of time and we aren't even close to done with this lesson. So I wrap up with a crappy little lecture about the importance of listening that I am sure sailed right out of their heads the second the bell rang.

Fortunately, I had a free period to reflect on this experience. 

Revised lesson plan

In my second and third Pre-Algebra classes, I explicitly pointed out this method of measurement, but complained about its imprecision. "This is the best we can do? Get out a ruler, measure the line, and then measure some blocks to estimate how long the line is? There's got to be a better way!"

That is a common refrain in my class. I do the whole infomercial voice and everything.

So then I hand out this sheet of paper, where the triangle has a square coming off its hypotenuse. I ask students to draw and shade in squares for the two legs.

We talk about how the small squares have an area of 25, which makes sense because the square root of 25 is 5, which matches the side lengths. So then if we knew the area of the big square, we could take the square root to find the length of the hypotenuse!

Then I get the kids to count the squares inside the big square. Most kids come up with something around 50, which leads to a side length of roughly 7.07. Pretty good thinking, E!

I wrap up class by saying "So that's how you find the long side of a triangle. You draw it on grid paper, then make a square from the long side, then count the area of that square, then take the square root of that area to find the length. Unless... there's a better way."

We'll be cutting out the small squares and fitting them into the big square like puzzle pieces tomorrow.

Reflection Questions:

1) Teachers make a lot of decisions throughout the day.  Sometimes we make so many it feels overwhelming.  When you think about today, what is a decision/teacher move you made that you are proud of?  What is one you are worried wasn’t ideal?

I am proud that I was able to incorporate E's idea into my subsequent lessons, even if her class didn't get that same experience. I'll be sure to bring them into the fold tomorrow.

My worst decision was not making 10 minutes to review my lesson at some point on Sunday night or Monday morning before I taught it. I need a fresh look at a lesson within 24 hours of when I teach it. Planning a lesson on Friday for Monday is fine, but only if I review it quickly on Sunday or Monday.

2) Every person’s life is full of highs and lows.  Share with us some of what that is like for a teacher.  What are you looking forward to?  What has been a challenge for you lately?

My challenge right now is managing all my responsibilities in school while also pursuing career goals outside of my classroom and being the primary parent. Here are my yearlong goals aside from "be a good 8th grade math teacher"

• Pilot a standards-based grading system with my 8th grade math colleagues
• Complete at least 2 components for National Board Certification
• Begin research on how kids comprehend expressions and equations for my Heinemann Fellowship
• Refine my integer unit before I present it at ACTM in November, and then refine it further and create a website to house the unit as a whole
• Brainstorm a daylong and a weeklong early math camp in the style of Math on a Stick and Talking Math With Your Kids
• Tutor a former student who is struggling in high school geometry.
• Keep my blog interesting, including with these posts

It's a lot. Something's probably going to fall by the wayside, and I don't know what.

3) We are reminded constantly of how relational teaching is.  As teachers we work to build relationships with our coworkers and students.  Describe a relational moment you had with someone recently.

I have a student who started the year with a pretty oppositional attitude to her teachers. On top of that, she has significant conceptual gaps in her math background. But she is a critical thinker and can make connections on her own when given enough support. 

So I have been giving her praise whenever it felt authentic to me, and meeting with her some one-on-one to give her some of the foundation she needs to succeed in my class. She is slowly warming up to me. 

4) Teachers are always working on improving, and often have specific goals for things to work on throughout a year.  What have you been doing to work toward your goal?  How do you feel you are doing?

I am still reading a lot. I have been working through some of the Transition to Algebra resources, which I really enjoy, and reading Connecting Arithmetic to Algebra by Russell, Schifter and Bastable. I will need to make a pre-assessment for expressions and equations by next month's post.

5) What else happened this month that you would like to share?

I am co-planning a lot with my colleagues, and I LOVE it. I love when I make a lesson that succeeds in my coworkers' classes, and I love using a lesson found or developed by a colleague that connects with my students. If I weren't coplanning, I wouldn't have even found the lesson that I screwed up today! But I am convinced it's the best way to introduce the Pythagorean Theorem that I've found.

A Sample Number Talk

In my last post, I wrote about my big-picture thoughts on Number Talks. Here's a post about the nitty-gritty.

Today in Pre-Algebra we are reviewing how to convert from fractions to decimals. I have this worksheet for all the fractions from halves to tenths. To solve this whole sheet, students will need to do some long division, so I chose a number talk that might bring up long division naturally as a strategy.

My Number Talk for today was: 

Find half of 36

At the beginning of the day, I anticipate the following strategies:

• Use long division (the traditional algorithm)
• Break 36 into 30 and 6, take half of each and add the halves back together to get 18
• 6*6 is 36, so 6*3 is 18. (Not certain, but possibly
• Who knows? I'm excited to see what they come up with

Here's how it went:

A Period

Billy started us off with long division, the traditional algorithm. I'm glad he brought it up and glad to get it out of the way!

Theo said "I added a 0 and got 360. I know 360 degrees is a full circle, so 180 is a half circle. Then I took away the 0 and got 18." This was unexpected but awesome! I asked for clarification from the class as to what we are doing mathematically when we "add or take away a zero" and talked about how we are multiplying or dividing by ten.

Elsa broke up 36 into 30 and 6 and found half of each, just as I anticipated. I reminded my students of the name for this strategy: decomposing 36. I like that term because it sounds gross and is memorable for that reason.

Billy said you could count by 2's until you got to 36. I wrote it down but kind of brushed it off. In retrospect, I should have dug deeper. Why does this strategy work? Why don't we count be 3s or 4s? If we did count by 3s, what would that find for us? What a missed opportunity!

Instead, I asked everyone to try Elsa's strategy on the number 74. I got a kid to explain it, and we realized that he not only decomposed 74 into 70+4, he then decomposed 70 into 60+10! So we had a double decomposition.

B Period

Ok, these guys rocked it. I had a total of 8 strategies for solving this problem! 

Karina said that 3/2 = 1.5 but she knew it was really 15, so I made another student explain that part. Anna Marie used a building-up strategy to find what added to itself to equal 30 and then 6. Above, you can see where I pointed out that both of them had decomposed 36 in the process of solving their problem.

Elizabeth Rae used a similar building-up strategy to Anna Marie, but with a twist. She said "I know 15+15 is 30, and I know that 8+8=16, so I figured out that 18+18=36" I asked her to expand on that, and she said she was using the units place to figure out that 8 and 8 make a number that ends in 6, and 18 is close to 15, so it made the most sense as an answer.

Landon started with 15 as well, but multiplied by 2 instead of adding it to itself. Then he guessed and checked until he found 18.

Jackson decomposed 36 into 20 and 16 instead of 30 and 6! What a great moment! I asked the question "So can we just decompose 36 into any pair of numbers?" and a kid said "As long as they add to 36, it should work." We will definitely need to revisit this in the future.

Reggie found a pattern with 6. 6*2 is 12, and when he added 6 to the first factor and tried 12*2 he got 24, so he added another 6 and tried 18*2 to get 36. I'm still not sure how he stumbled on this idea, but it's cool! I hope he brings it up again in the future.

Jeremy decided to divide 36 by 4, since he knew that math fact. The he multiplied than answer, 9, by 2 to get 18. I asked another student to explain why this work, and I used a visual model (at the top of the picture) to solidify the explanation.

Kayla used the traditional algorithm! Finally!

C Period

This period we had our first controversy! One student got 13.5 and another got 18. All last week, we had controversy every day, so I was actualy surprised that we hadn't had disagreement in A or B period. I always get excited when a student voices a wrong answer because they might be brave enough to share their thought process and explain their mistake.

Anna Lane decomposed the problem in the way I anticipated, into 30 and 6. Haley used the traditional algorithm.

Grant did the strategy I was anticipating but unsure of, where he knew that 6*6 is 36, so 3*6 is 18. I asked him "Why not cut both numbers in half?" And he said "that wouldn't work." And I said "why?" and it got a little confused but eventually someone said "because 6*6 means you are counting six 6s, so Grant is only counting three 6s. So that's half"

After Haley did the traditional algorithm, Macon said "I see what I did wrong. I did long division, and I knew that 2 goes into 3 once and 2 goes into 6 three times. So I got 13. THen I knew I had a remainder of 1 from the 3/2, so I brought down a zero and did 10/2 and got 5, which I added at the end as 0.5. But I needed to bring down the 6 and do 16/2"

I then pointed out that his idea was almost right! He divided 30 by 2, not 3, so that 0.5 should be in the units place, where it would add with 3 to get 8. Then he would have gotten 18. This is where I wish I had my Great Mistakes board up so I could give the class a point for Macon's great mistake.

What I Learned Today

• I can anticipate the most common strategies, but some (like Theo's or Reggie's or Jeremy's) will be totally out of left field and surprise me
• Until this year, I can't think of a single time I've had 8 students share 8 different ways to solve a single problem. B Period was on fire. And we built another bit of classroom culture that the more ideas, the better.
• Macon is brave.
• I need to pull back my participation. I did a couple of moves where I said "someone else explain that to me" but in writing this blog, I realize how many times I added something that I didn't need to add.
• I like the idea of "Try Elsa's strategy on a new problem" as a good way to extend the conversation if there aren't a ton of different strategies. 
• The same kids keep sharing. I think I am going to grab a couple of shy kids on Monday and say "I would love for you to share one method in our Number Talks this week. Raise your hand on a day when you feel comfortable sharing"
• In a month or so, when I introduce the distributive property, these kids are going to roll their eyes at how obvious it is. I think I will slowly formalize my notation until I write these problems in a way that looks more and more like the distributive property.

Number Talks

Seven days in, and I am sold on Number Talks.

I had been hearing about Number Talks for a couple of years, but it was finally Megan Schmidt's persistent updates on Twitter that made me try them in earnest this year.

If you haven't heard of Number Talks, read this book. But if you want a short summary, here is how a number talk works:

• Put an arithmetic problem, such as 111 - 29, on the board
• Students place a fist on their chests and raise their thumb when they have an answer (and other fingers when they have multiple strategies)
• Record all student answers without indicating the correct answer
• Ask for volunteers to defend an answer and explain how they solved the problem. The teacher tries to take notes on the board that replicate the student's thought process
• Ask for more and more, never giving away the correct answer. It will become clear to the students anyway
• Pick something cool or interesting from a student strategy and highlight it or ask a question about it.

So far, my goal has not been mathematical, but instead to model and promote the sort of mathematical conversations I want to hear in my class. But because my students are awesome, we got some great mathematical conversations anyway!

For example, in one class a student solved 111 - 29 by "sliding" the problem up by one, up to 112 - 30. I asked for another student to explain the strategy and then modeled it using a number line.

It became a favorite strategy all week for kids, until Friday when a student used the method to slide 5.1 - 0.35 down to 5 - 0.34. This promoted a GREAT conversation about why we need to be precise with our language in math class. Kids had been saying "five point one" or "point thirty five" but then switched to "five and one tenth" after this conversation. I think this will be a more memorable lesson because it came up in the context of a mental math problem that students had thought deeply about.

I will probably start recording some of these talks and transcribing the best bits for future blog posts. Seriously, I wish I had a court stenographer in my room so I could just show you guys how great the conversations have been already. 

Also I have a big idea about using Number Talks to teach about the properties and how to manipulate algebraic expressions, but that's for another day. For now:

My tips for starting Number Talks

1) Read Making Number Talks Matter. Seriously, it is such a good book. It's full of great classroom tips to promote and deepen student thinking using the Number Talks structure.

2) Start with dot pictures. This is a tip straight from the book, and it's great. I did three days of dot pictures, which helped set a norm that I expect lots of answers and lots of participation. On the first day I used this picture:

And just look how many ways my students saw this answer:

Even Mr. Taylor, my inclusion co-teacher, got in on the action!

3) Have the kids stand up. I think it sets a tone that this is using your brain in a different and more active way than most warm-ups. Besides, kids have fewer distractions when they stand.

4) One idea I haven't started yet is to have a Great Mistakes board to provide an incentive for students to share their ideas, even if they may be wrong. The idea is to give each class points whenever a student makes a mistake that leads to a valuable learning moment, such as the place value error in the decimal problem above. Extra points for a student who bravely shares something they did wrong and explains why it was wrong or how to correct it. When the class gets to 10 (or 20 or whatever) points, the whole class gets a prize. Our school is trying a positive behavioral support system that I might try to tie into my Great Mistakes board.

Good luck! Let me know how it goes!

Math Autobiographies

“Dear Math:

I hate you.”

— J

This year, I did the best getting-to-know-you activity I’ve ever done. I had my students write math autobiographies, telling me their personal history with math, warts and all. And two days into school, I know more about my students than I knew after 4 weeks last year.

“I used to hate math, but since the problems got more challenging I have started to love it more”

— E

Here’s how I did it: On the very first day of school, I took 5 minutes to introduce the idea of a math autobiography and ask students to brainstorm their own for homework. Then i read my own, which I’ve reprinted below.

“Math always came easy to me. I think that’s because as a kid I used to love finding math patterns wherever I went. I would always like playing with shapes, finding out which ones went together and trying to figure out why. I would count the number of stairs in my house and the number of tiles in the ceiling of my elementary school classrooms. On long road trips I would occupy my mind by turning each number into a number based on its place in the alphabet, (A=1, B=2, C=3 and so on) and then adding all the numbers to try to find words that added to 100. I know how nerdy this sounds, but when I was a kid I thought everyone just played math games in their heads.

But math class was always very boring to me. It was my least favorite part of school. I memorized the algorithms my teachers taught and tried to finish my homework as quickly as humanly possible. Honestly, I don’t even remember my middle school math teachers’ names because I never had a single interesting day in their math classes.

But finally in 11th grade I started to see how math could be interesting. I had a teacher, Dr. LaCasse, who taught my calculus and physics classes the same year. He did a fantastic job of combining those subjects so that I could see how I could use calculus to model a situation in the real world, or predict the speed of a person who jumps out of a plane or the distance that a bowling ball would fly if you shot it out of a cannon. Finally, math was real to me.

My favorite topic was always geometry. I loved using a compass and a ruler to make shapes and beautiful designs. I think geometry is the subject that shows off how beautiful math can be. Also, I love logic games like Sudoku, and lots of geometry problems felt like mini logic puzzles for me to solve. Even calculus and physics involved drawing a lot of geometric models of things like pulleys and ramps, which I loved.

Since becoming a math teacher, my relationship with math has totally changed. I love talking about math and thinking about it. But I hate teaching by just giving kids steps to memorize. Instead, I want everyone in class to be thinking for themselves and finding their own solutions to problems, using the skills they learn in class. And my favorite part about math is that I still learn new strategies and ways to solve problems from my students. Every year, I see some student work that just blows my mind with how cool and creative it is. And I can’t wait to see what you guys are going to show me this year.”

— Mr. Haines

The next day, I read a very different autobiography from my colleague, Mrs. G, who teaches Language Arts to all my students. Here is her essay.
 

“I have a love/hate relationship with math. If I were to write a love letter to math, it would sound like this.
Dear Math,
You’re so cool. You’re so cool that I can’t figure you out. I know deep down that you are awesome, but you’re too far from reach. We can never truly be together.
Love,
Elena

When it came to working through math problems in school, I thought I was a great student. When it came to math homework, I followed directions. I checked my work. I worked the problems just like the examples we did in class. When we went over the problems the next day, I figured out why they’re called math PROBLEMS—because there was always a problem with the way I worked them.

The only math teacher that ever seemed to understand my love/hate relationship with math was Mrs. Llewellyn, my Algebra II teacher. She could see the way my brain followed the path to the wrong answer, then she would show me the yellow brick road. She would explain in words I understood how to correctly make it to Oz.

I don’t remember my least favorite math teacher’s name because she was that awful. I’ve tried to erase her from time and space. She always called on me to work problems at the board. The pressure made me so nervous that I would miss a step in solving the equation. Then she would make me stand at the board until I figured it out. Sometimes I never did, and it was really awkward. She also yelled all the time. “TWO NEGATIVES MAKE A POSITIVE!!!!!!!” I still don’t understand that whole concept. Two negatives should make something more negative. Am I right??

The only math that I kind of understood was geometry. To me, the shapes had rules that made sense. The angles were always the same. They didn’t change on you like those tricky negative signs. Also, geometry is where I learned about how letters can be used in math. And I love letters. With all the shapes and the letters, geometry is really more like English—the best subject ever.

I wish I knew how do work math quickly in my head, but I can’t. I still have to get out a calculator or use my fingers to count the 9s times tables. That’s probably really pathetic for a grown up, but I get so nervous that I’m going to mess that I just check myself with a calculator before I make a mistake and have to stand at the board until I fix it.
”

— Mrs. G

I love this essay because it feels honest to me, unlike so much of the motivational stuff that teachers say to students on the first days of school. After I read Mrs. G’s essay out loud, I told my students how much I appreciated her honesty. I told them that all I wanted was their honesty as well. If they love math, tell me why. If they hate it, tell me why. 

Then I gave out the prompt below. I didn’t ask for a specific length. I just asked everyone to address all six questions:

“Instructions: You will write your math autobiography on a separate sheet of paper. In your autobiography, be sure to include the following:

Do you like math? Why or why not?
What sort of math student do you think you are? Why?
Who was your favorite math teacher? Why was that person your favorite?
Who was your least favorite math teacher? Why was that person your least favorite?
What math subjects do you like learning about and why do you like them?
What math subjects do you dislike and why?”

Most kids wrote about 3/4 of a page, and I had to cut a lot of kids off after 20 minutes! I never expected so much engagement and enthusiasm from a getting-to-know-you activity.

“I can do most math problems but I don’t understand them. I get all As and I do my homework. I just don’t understand math”

— A

What Next?

I asked the kids to be honest, and man, they were. I have a few kids who really seem to love math, but most of my students have had a pretty negative math experience so far. Honestly, I felt a little bit like a trauma counselor reading these. I saw so many stories of teachers yelling at students or shaming them for making mistakes.

“It’s like math for me is something in a B-flick horror movie. You think you got away but then you somehow trip for the third time.”

— M

I went through each autobiography and made sure to underline and comment on at least one sentence or phrase. I handed the essays back today so that my students know that I read their work. I feel like I asked a lot of them, expecting them to open up to a stranger on the second day of school. I owe them at least a comment.

“In third grade my teacher had a happy meter from 5 to 0. Whenever I messed up a problem she would bump me down a number.”

— D

In fact, I wish I had time to write an essay back to each kid. I already feel more connected to my students than I usually do after one month of teaching. I have a wealth of personal stories that I can connect to each student in my room. I now feel even more committed to making my class a model of engaging, challenging mathematics and conversation.

“Math is my favorite subject because to me it is more challenging than the other classes.”

— E

I can't recommend these autobiographies highly enough. If you're wondering what to do on the first days of school, try this out. You might be surprised what your students write to you.

Below are a few more quotes from my students, which I also threaded throughout this post.

“I’m really in between a weak and a medium student because every time I get confused I get headaches and give up.”

— I

“I like math, but I’m not sure that math likes me”

— J

“I always feel like I’m doing something wrong but I end up getting a good grade. It’s not a good feeling b/c I don’t know what I did right”

— L

“So am I good at math? Yes and no. So many hidden questions in that one question.”

— M

Day in the Life - August 12th

I am contributing to Tina Cardone's Day in the Life series this year. I'll be writing about the 12th of each month.

Today was the second day of school, which is like the first day of school but better because there is less chaos and also it's Friday.

Today I got to school around 7:30, drinking my second large coffee of the day, and ran through my lesson. Here is my day:

1st: Pre-Algebra

2nd: Free

3rd: Pre-Algebra

4th: Pre-Algebra

5th: Algebra 1

6th: Academic Strategies (study hall)

7th: Free

Last year I had 1st period off which felt great but was bad for me. I'd spend that period prepping for the day's lesson, so it gave me an excuse to slack off the night before. I much prefer this year, where my last period is free. That gives me a chance to prep for tomorrow (or write a blog post about today)

I started all of my classes with a Number Talk. I am using the approach recommended in Making Number Talks Matter, and so far it's going well. We are just counting dots so far, but the kids are getting used to explaining their thought process and offering multiple solutions, which is exciting. We will move on to arithmetic next week at some point, but for now I just want to hear as many voices as possible

The main task we did in class today was a math autobiography. Yesterday I read my own to the students, and today I shared my colleague's autobiography. She is a Language Arts teacher with a math phobia, so it was great to get the kids to hear two very different autobiographies. I told the kids that the most important thing is that they are honest.

And boy, were they! This is without a doubt the best getting-to-know-you activity that I've ever done. I had to cut kids off after 20 minutes of writing and some kids were upset they couldn't write more! I mostly got a full page from each kid, even (especially) those who struggle in math and hate it. A lot of the stuff I heard is the sort of stuff that would take weeks or even months to find out in class. Some telling quotes:

"I can do most math problems but I don't understand them. I get all As and I do my homework. I just don't understand math"

"I like math, I'm just not sure that math likes me"

"I'm really in between a weak and a medium student because every time I get confused I get headaches and give up"

"I used to hate math, but since the problems got more challenging I have started to love it more"

I could be here all day, quoting from every single one of my 80+ autobiographies.

For most of my classes, I ended the day with the Pitcher Problem: You have a 5 gallon pitcher, a 3 gallon pitcher, and an unlimited supply of water. Using only these things, how can you get exactly 4 gallons of water?

Nobody solved it in class, but we will revisit it on Monday and work it through. The goal is to promote discussion and group work, so I am going to structure our discussion on Monday around tips for productive collaboration.

We didn't get to this in Algebra 1 because our class is split in the middle by lunch, which I HATE. I seriously can't stand getting interrupted in the middle of a class, or having to make sure my lesson has a clean break 20 minutes in every day. The struggle is real.

In my study hall, I only have 2 students, which is silly. There is nothing worse that the awkward silence that settles in on 2 or 3 middle school students who are stuck in a room with a teacher, especially when it's the second day of school and they have no homework to do. I might just go join a new class with my 3 muskateers.

Reflection Questions:

1) Teachers make a lot of decisions throughout the day.  Sometimes we make so many it feels overwhelming.  When you think about today, what is a decision/teacher move you made that you are proud of?  What is one you are worried wasn’t ideal?

One thing I did this year was really try to find ways to start a conversation with my students, especially those that seem a bit hard to reach at first. I know I would never have thought to do this in my first year. I was so overwhelmed with the work of teaching that I ignored the whole aspect of making relationships until much later.

But now I know that relationships are the absolute most important element of being a middle school teacher. If I get that part right, everything els gets much more manageable.

2) Every person’s life is full of highs and lows.  Share with us some of what that is like for a teacher.  What are you looking forward to?  What has been a challenge for you lately?

I am on such a high. I actually have decided that I hate the summer. Or rather, it is far, far too long. I wish summer were only 3 weeks long. Then I could recharge and rest without feeling terrible about wasting so much of my free time. With a 9 week summer, I have all the ambitions to complete these enormous projects, but not the structure that a school setting provides for actually getting things done.

I get a little depressed in the summer because I just don't feel as useful if I'm not teaching. I feel like an athlete who is on bed rest. I need to teach for me, not just for the kids.

3) We are reminded constantly of how relational teaching is.  As teachers we work to build relationships with our coworkers and students.  Describe a relational moment you had with someone recently.

I have a poster in my room that says "Math is Beautiful" and has a piece of mathematical artwork I made. I'm not much of a freehand artist, but I can wield a ruler and compass pretty well. I noticed a really quiet kid doodling in my class yesterday, so I told him that if he made any mathematical artwork I'd be happy to add it to the board. He's really opened up to me today. 

4) Teachers are always working on improving, and often have specific goals for things to work on throughout a year.  
First post: What is a goal you have for the year?  

My goal this year is to totally reorient my teaching of expressions and equations. I HATE the way I taught expressions and equations last year, where we combine like terms for a couple of days and then add in the distributive property and then negative coefficients and it's weeks and weeks without any big-picture conversation about what this all means. 

So I am trying to approach expressions and equations from a literacy angle, meaning that I want students to be able to read an expression and understand what it means before they try to manipulate it into a simplified form or a solved equation.

I have some beginning ideas about how to do that, but they haven't cohered yet.

5) What else happened this month that you would like to share?

Not much, since I wasn't in school yet. I moved, but moving is one of those things that is simultaneously terrible to experience and boring to talk about, so I'll leave it there.

Algebraic Literacy

More than ever, I need the guidance of the Math Twitter Blogosphere. 

Background: I got a fellowship from Heinemann to do some action research on a topic of my choosing over the next two years. I am trying to wrap my head around a big, complicated topic and I’d love anyone’s input. There’s something I want to research, and I don’t know what it’s called, so I’m calling it algebraic literacy.

Here’s what I mean: When we ask students to read a passage in language arts class, we certainly expect them to be able to read each word in a given sentence. But we also expect them to be able to summarize the content of the sentence as a whole. Moreover, we hope that they can mentally break the sentence into its phrases and clauses, knowing which noun is being modified by that adjective, or which clauses are independent or dependent. These skills are a significant part of our students’ growing levels of literacy.

But in math class, we have a less refined understanding of algebraic literacy. Let me give you a context and a matching expression so we have something concrete to discuss:

Ava has a job at a restaurant that pays her a wage of w dollars per hour. One day, Ava's boss informs her that she is getting a $0.50 raise on her wage. That night, Ava works for 7 hours and also earns $43 in tips.

Here’s the expression:

7(w + 0.50) + 43

Kids can read the expression above on a number of levels. At a baseline, one could read the expression phonetically. Lots of my kids can do this. They can say “seven times w plus point five plus forty three.” 

On another level, kids can read it word-by-word and also understand its mathematical structure for purposes of evaluating the expression. Meaning, a student could substitute in a given value for w and evaluate using the correct steps.

On yet another level, kids can read the expression and understand how it represents the context of Ava’s job. On this level, kids could identify that the entire expression represents Ava’s total wages. Beyond that, kids could match specific pieces of the expression with their corresponding parts of the context. For example, if asked “Find the part of the expression that represents Ava’s new wage” students could identify “w + 0.50” as that element.

This is the level of algebraic literacy I want to focus on. Kids have a REALLY hard time generating expressions from a given context, and I think it’s because we focus too much on the phonics of algebra and not enough on comprehension. 

So that’s what I want to study. But I need to answer a lot of questions first. Questions such as:

1) I am calling this “algebraic literacy” but I am sure that other people have researched it and called it by other names. Where is this prior research and what are people calling it?

2) How do kids attain higher levels of literacy in English, and to what extent is that trajectory transferrable to algebraic expressions and equations?

3) What tasks, activities and structures would be most useful for helping me understand my students’ current levels of algebraic literacy? Which structures would most facilitate their growth in this area?

4) There’s another level of literacy that I can’t quite wrap my head around. It’s the literacy around the idea of equivalence. I want kids to know which sorts of algebraic manipulations maintain equivalence and which affect the meaning of the expression or equation being manipulated.

As an analogy, think of the sentence “Tom and Joey gave Denise an apple"

A language arts teacher would want kids to understand that switching Tom and Joey in the sentence preserves the meaning, whereas switching Tom and Denise in the sentence changes something fundamental about the problem.

In the same way, taking the expression 14 - 6 + 5, I want kids to know why we can switch this to 14 + 5 - 6, but not to 6 - 14 + 5

There’s a lot going on in this post. Let me know in the comments - what resources does this make you think of? 

Uncle Wiggily

If you follow me on Twitter, you know I am a devoted acolyte of Christopher Danielson and his Talking Math With Your Kids ethos. I truly love teaching middle schoolers, but nothing compares to the joy I get from talking with young children about how they perceive numbers, shapes, and patterns.

A couple of years ago, Christopher wrote a great post about the board game Uncle Wiggily, which I promptly ordered online. It sat on the shelf until my son Joel, who is 3 1/2, asked to play it. 

And so we played Uncle Wiggily. And then we played it again the next night, and the next. And we have continued to play it five or six nights a week for the past three months. My wife and I may not always read to Joel for 20 minutes a night, but we definitely play Uncle Wiggily for 20 minutes a night.

As you might guess, I got bored with playing Uncle Wiggily much faster than Joel did. It is a game that is entirely based on luck, which means there are no decisions to make during the game. So to stave off boredom, I have found questions and minigames to play with Joel. I realized that they provide a good roadmap for how I talk math with my own kids. 

---

Uncle Wiggily has been a wonderful way to help Joel develop one-to-one correspondence (the idea that each number corresponds to one item in a group). In the game, you hop your bunny along the path according to the number listed on the card. 

He was pretty good at counting objects in groups of ten or less, but he was less comfortable counting spaces. He would often speed up his counting so that he would count to ten but only hop seven or eight spaces. 

So I tried to mess with him by taking a cue from Christopher and deliberately messing up, seeing if he would catch me. But I was much less subtle than Christopher. I would draw a three and then jump seven spaces at a time. Or I would draw twelve and only hop a couple of spaces while I raced through the numbers as fast as possible like "onetwothreefourfivesix…"

Joel loved acting as the Uncle Wiggily police and showing me how to play the game correctly. He found it very funny, which showed me that he was beginning to understand the importance of one-to-one correspondence. This sort of violation of the rules is only funny if he knows which rules are being violated.

Having gotten bored with counting and miscounting, I started asking Joel to compare cards, as in the videos below:

This was a fun extension because the game gave Joel a reason to care about the relative size of the numbers. By playing the game over and over, he got a sense of which cards allowed him to move farther along the path to Uncle Wiggily’s house. If I had shown him the numbers 7 and 4, I don’t know whether he could have told me which number was greater. But in the context of the game, he has a reason to care which is greater. The math is embedded in the game.

Notice in this video how he doesn’t have a complete understanding of the difference between 8 and 9. But he knows how they relate to 7, 5, and 1. It’s really interesting to me to see those ideas as they begin to cohere. Also note that I have followed Christopher’s advice and chosen not to correct every little mistake he makes. I am much more interested in the overall idea of comparison that Joel is working on.

But then Joel got bored with this game, and it kind of interrupted the flow of Uncle Wiggily, so I needed a new activity.

---

Space 58 on the Uncle Wiggily game board is a very important space. It’s the Rabbit Hole, which automatically sends the lucky rabbit all the way to space 83. Consequently, Joel spends most of the game talking about how he hopes he lands on the rabbit hole. So once he got to space 45 or 50, I started asking him “What card will get you to the rabbit hole?"

This is a bit of a reversal of the normal game because Joel has to count first and then decide which card will get him to the rabbit hole. If he counts five spaces, he knows he needs a five (this is known as cardinality, the idea that the last number you count to represents the total group).

Joel liked this idea so much that he now checks every round when he is close to the rabbit hole to see which card will transport him to space 83. Nothing has made me happier than to see him count out five spaces, say “I hope I get a five!” as he draws the card, draw a six, and then glumly move his bunny directly to space 59. This is the only time I've ever seen him move his bunny directly to the correct spot, by the way. 

---

One night, I picked my card but didn’t show it to Joel. Instead, I told him to guess. Joel loved this game, and insisted we play often. It really slowed down the game, but it was worth it. 

Let’s say I draw a three. Joel guesses “Ten!” and I say “Lower!” Then he says “eight!” and I say “Lower!” 

We continue on like this until he gets the right number. Joel liked this game so much he asked if he could be the picker and I could be the guesser. I have a video of our first or second round:

(Joel’s rash is from a penicillin allergy. He stayed home from school for a day and we played Uncle Wiggily. A lot.)

Notice that Joel is much more confident earlier on in the game than in the last couple of rounds. This game requires a much stronger sense of ordinality (the relative value of numbers) than simply picking whether seven is greater than four. 

(Watching this video again, I realized that I always move in the direction that Joel indicates. I wonder what Joel would do if I said "four," he said "higher..." and I said "three!" Now I have a new question to try out next time we play.)

One big idea I've gotten from Natural Math's wonderful books is that any child can engage with any math idea at some developmentally meaningful level. So naturally I wanted to see whether Joel could grapple with integers!

One night, I drew the dreaded “Go Back Three” card. I asked Joel to guess what it was.

As he neared one, his shouted numbers got more confident.

Three!

Lower...

Two!

Lower...

ONE!

Lower...

...

Joel paused for a moment, then looked at me and said “Go back two!"

I looked at him despondently and said “Even lower…"

Another, shorter, pause.

"Go back three!"

---

As Joel has gotten better at one-to-one correspondence, he has been less reliant on counting out loud. Sometimes he makes noises instead of counting, and sometimes he says nothing at all, as in the video below.

Joel called this “counting without counting.” I don’t know the name for it in early math literature, but it’s pretty cool. He can do this with numbers up to about 5 or 6. His favorite is to yell five nonsense syllables while moving five spaces. Really, he takes any opportunity to yell nonsense.

---

I still have a couple of ideas for how to continue exploring new math ideas with Joel using Uncle Wiggily. Aside from the idea I had above, my big new idea is to replace the game cards with a pair of dice. I feel like there is a lot of great conversation to be had about subitizing, adding, and commutativity. Also, I have thought about playing a variant on the game where we each draw 3 cards at the start of the game and choose which card to play. 

I hope this blog post gives a sense of the ways that I’ve tried to guide Joel into new mathematical territory by finding interesting questions within a game that he already loves. Three year olds love routine and repetition, so I think it's useful to harness that natural desire and aim it in a mathematical direction. 

And man, does Joel love repetition. I don’t always love playing Uncle Wiggily with Joel, but I do like those nights when I see him stumble on a new idea. Since we play almost every night, I don’t have to worry about pushing him to new ideas every day. Sometimes I can just sit back and let him play around with the math ideas we’ve discussed over the least week or so. And sometimes the lesson of the night is more about winning and losing with dignity than it is about math. Joel reaalllly likes to be the first person to 100 (probably because to him, everything is out of 100). 

Everything Out of 100

My son J, who is 3 1/2 years old, has an adorable habit of rating everything out of 100. A building in downtown Birmingham is 100 tall, and a watermelon is 100 heavy. At one point, we were playing by a creek and he said “to go from here to the other end of the creek would be 100 swimming"

Since I can’t help myself, I’ve been theorizing about how he has come up with this habit. 

There are two contexts where J hears numbers used without units as a way to measure something. The first is age. I don’t typically say “I am 30 years old.” I just say “I am 30.” So J knows that he is 3 1/2, his sister is 1, and his parents are 30. Sometimes he hears us say that 30 means 30 years, but he still doesn’t have a firm idea of what a year is, so to him it’s likely just an arbitrary measure of age.

The other context where he hears number without units is driving speed. He sees speed limit signs that say 25 (which he sometimes reads as fifty two) or 60 or 70, and when I talk about driving I usually say something like “I am going 55” as opposed to “I am going 55 miles per hour.” Again, even if I used the term “miles per hour” J would have no context for understanding that unit of measure. So mostly I just say “I am going 30 now, but on the highway I will go 70.” In both cases, J hears grown-ups using unitless numbers to measure a quantity.

Moreover, in both cases adults are talking about measurements (years and mph) where 100 denotes an extreme amount. When J asks me about someone who “is 100,” I tell him that a person who is 100 would be very, very old. This connects with his existing idea that 100 is a very big number. Similarly, he often asks me to “go 100” in the car. I tell him that going 100 is very, very fast, and in fact I’ve never gone 100 because it is so fast. So again, J reasonably can conclude that 100 represents the extreme measurement of speed.

So now everything can be rated out of 100! Whether it’s height, weight, or swimmingness, J can use 100 as his benchmark.

I don’t really know what to conclude from this mental exercise I’ve taken myself on, other than to say that I think that kids come up with the ideas they do for a reason, and I think it’s fun to try to figure out what that reason might be. 

I truly love teaching middle schoolers. But nothing has been as fun to me as watching a kid develop his very first mathematical ideas. I’m so excited that I have so many years of math conversations in my future, both with J and his sister. 

The Power of Zero

I don't lecture in my class a lot, but today I did. 

I have an explanation for zero as an exponent that I like a lot. I've been tweaking it for a few years. It's similar to a lot of other explanations, in that it explores the patterns of powers, but there are some subtle differences that I think kids really connect with.

I've been meaning to try out the iPad app Educreations for a while, so I figured I'd record my mini-lecture as a test. 

Check it out and let me know whether you can predict how I incorporate negative exponents into my next lesson!

Test Run on Standards-Based Grading

I have amazing colleagues in 8th grade math. In the fall, we were complaining about how little our grades communicated to students and parents about each student's learning. So we decided to build a standards-based grading system for next year.

But we don't just want to dive in headfirst. We want to test it out this year. That way we can decide from experience what aspects of our SBG system work well and which aspects need tweaking.

So I wrote a quiz for my pre-algebra students and also wrote a set of standards that I felt my quiz was assessing. Then my colleagues and I sat down and graded a bunch of the quizzes using this rubric.

First, the good news.

1) My colleagues and I were generally in agreement about how to grade student work. I was worried that we would have wildly different opinions about what constituted a 2 vs a 3, but it turns out we are in agreement most of the time!

2) I like the rubric, generally. I think the explanations of each rung on the ladder of mastery make sense to me. I might have some quibbles with the conversion to percentages or the Level 5 standard, though. In my view, Level 5 should be something like "You can apply the concepts of this standard to new and unfamiliar problem types" or something like that.

3) It didn't take me much longer than usual to grade the tests. Since I hand-grade every test and only include open-response questions on my quizzes, grading takes me a while anyway. So this didn't add much time to my grading. My colleagues to somewhat longer because they didn't write the quiz so they had to work or re-work the problems to check student answers.

Now, the bad news.

1) Our school requires some form of percentage grading at the end of the year. That means we'll have to convert standard mastery into a number grade at some point. There are a bunch of ways to do this, but they are all predicated on weighting every standard equally. In my opinion, not every standard we teach is equally important to student success or numeracy. So there will always be that tension when writing the standards. Do you break a more important standard into substandards so that its weight is appropriate, given its importance to future success in math? Or do you just write all the standards without this consideration in mind?

2) I tried to write my standards as "I can" statements so that I could easily assess them. Now I feel that this was a mistake. First of all, there was this question on the test:

A line is drawn through the points (5, 8) and (9, 10). List another point that is also on that line.

I always have a question or two like this on my tests - something I never explicitly taught in class, but which can be solved by applying the concepts we've learned in class. This might be a Depth of Knowledge Level 2 question, but baaaarely. Only because I never taught a problem like this in class.

As a result, I don't want this to be a standard in my grading, or at least not an "I can" standard. If I include something like "I can find additional points on a line, given two points or a point and a slope" then I have to teach that explicitly in my class or else it's unfair to include on the test.

My point is, these standards, as I wrote them, are oriented toward answer-getting instead of conceptual understanding. And I worry that much of standards-based grading encourages students toward answer-getting. It's a much more communicative grading system, but it's still about "Can you solve this type of problem? What about this one? What about this one?"

So I tried rewriting my standards as "I understand" statements, such as "I understand the relationship between an equation for a function and its graph"

The best thing I came up with for my challenge problem: "I understand the relationship between the slope of a line and the points along that line." 

I am not very happy with that language. And I also think that this sort of "I understand" framework would lead to a lot more disagreement between my colleagues and me. 

On the positive side, if I changed everything to "I understand" then I could change that "5" category in the rubric to something closer to "5 - I can apply this concept to unfamiliar and non-routine problems." This would help me reach my overall goal as a teacher, which is to set the standard that 100% indicates more than procedural fluency on routine problems.

3) This process has helped me to see some of the drawbacks of standards-based grading, such as the ugly conversion to percentage grades or the tendency to focus the standards around answer-getting rather than conceptual understanding. 

So I emerge from this process more skeptical of standards-based grading. But I am EVEN more skeptical of my current grading system. Yes, there are flaws in any grading system. But every time I encountered a flaw in standards-based grading, I thought "Well, does my current system address that problem any better?" The answer was usually no. 

So now I feel obligated to move to an imperfect grading system next year because it's a lot less flawed than my current system. I don't think I can keep grading strictly on a percentage-of-questions-right model after this year. It's too blunt a tool.

Now I just have to make a comprehensive list of standards for both of my classes, find or create DOK level 2 or 3 problems for each of those standards, create a procedure for assessment, remediation and re-assessment, make some form of document or video explaining my system to students and parents, find a computer program that easily logs and communicates each student's progress through the standards, and decide how my school's required semester exams should be incorporated in this system. Piece of cake.

Draw Something Different

Years ago, I saw a stand-up show by the comedian Greg Behrendt. There's this one joke that I think about a lot.

He talks about the frustration of playing Pictionary with his wife. He gets a card, and it says that he has to draw the word "dry." He has no idea what to do, so he draws a bunch of waves and raindrops with a big X through them.

His wife says something like "No water!" and that's close, so he nods, but then she just keeps saying "No water! No water!" and he's stuck.

So then she starts yelling at him "Draw something different! Draw something different!"

So what does he do? He draws the exact same thing, HARDER.

This round of Pictionary does not end well.

I think about this joke a lot because I have a bunch of students in some form of math remediation. They tested poorly on fractions, so they are in a class to help them strengthen their skills in fractions.

The problem is, much of this remediation is just a slowed-down version of the exact same teaching methods that were ineffective for these students in the first place.

Their fifth grade teacher told them "To add fractions with different denominators, first you must find a common denominator and then convert each fraction to an equivalent fraction with that common denominator. Then you may add the numerators."

And now their eighth grade math remediation teacher (usually me) is telling them "To add fractions... with different denominators...first you find a common denominator... repeat that back to me, common denominator...then convert each fraction to an equivalent fraction..."

In other words, we are drawing the exact same thing, HARDER.

I think this type of remediation is ineffective because it assumes that the students just need to refresh their procedures and practice, practice, practice. But I don't think that these students are bad at adding fractions because they have been given insufficient opportunities to practice. In fact, I would say that procedural practice is something they've probably gotten too much of.

These students are the ones who can't get by on memorizing rules. They have to understand the concept of what a fraction is before they can manipulate it arithmetically. And one thing that is clear to me is that most students, even the ones who can add and multiply fractions, do not fully understand what a fraction is. I have many students in my class who can consistently add, subtract, multiply, and divide fractions, but cannot place the fraction 2/5 on a number line, or tell me whether 3/5 or 4/9 is greater.

I think it's time we draw something different.

Integer Solitaire

Note: This is the first math game I ever invented. In the years since, I’ve become obsessed with math games. I started a weekly newsletter, Games for Young Minds, for parents of young kids who are looking for math games to play together. Check it out!

This past week I finally presented my integer lesson progression to math teachers in my district. It felt good to finally get a room full of people to grapple with the same topic I've been working on for most of this school year.

I'll be posting my lesson progression over the course of the next few weeks, but I decided to go ahead and share a little game that I use to practice fluency at the end of the unit. You can also use this with 8th graders and high schoolers who need integer practice but don't want to do yet another Kuta Software worksheet.

I call the game Integer Solitaire, but it can be played alone or in pairs. All you need is some poster board or big white boards and decks of cards.

The Rules

Give each kid a poster board or a big white board. The board needs to be laid out like this:

Kids, working alone or in pairs, should draw 18 cards at random from the deck. 

Black cards are positive.   Red cards are negative

Ace = 1    Jack = 11      Queen = 12     King = 13

Kids need to use these 18 cards to somehow fill in the 14 blanks on their board to make 4 correct equations.

So a completed board would look like 

Things to keep in mind:

1) As kids try to fill out their board, they are mentally testing dozens of addition and subtraction problems as they go. Sometimes kids will get three correct equations, only to realize they can't make a correct fourth equation and they have to destroy and rebuild their entire board. If you gave kids this many problems on a worksheet, they would revolt. But they persist because they want to win the game!

2) The colored cards are, in some ways, even more abstract representations of integers than numbers like 4 and -3. Looking at a red queen and thinking -12 is a leap, which is why this is a good end-of-unit activity as opposed to a beginning-of-unit activity.  If you wanted to lower the barrier to entry, you could print out slips of paper with integers printed on them.

3) If a kid or a pair of kids completes the activity, tell them "Great work! Ok, now shuffle and deal yourself 17 cards and try again." I have seen kids complete this activity with 16 cards.

I love this game because it doesn't require a lot of supplies, can be played in 15 minutes, and remains challenging even after a student has mastered integer addition and subtraction. Please play with your students and let me know how it goes!

Extra Credit: I picked the starting amount of cards on intuition. I have no idea whether all combinations of 18 cards are solvable in this game. But I have played this game for five years with dozens of students, and I have yet to see a combination of 18 cards that is unsolvable. Even so, I don't know how to prove that 18 cards is always solvable. Any ideas? 

Thoughts on "On Visual Patterns and Feedback"

I wish I knew how to quit Michael Pershan.

As readers may remember, we collaborated on a series of blog posts about integer operations which led me to develop a unit of instruction and then present it to my whole district this past Monday and Tuesday (More on that in future posts).

So on Wednesday I'm riding high, and I walk into my colleague Amy's room and say "You know what I did with integers this year, where I fleshed out a whole unit of instruction? I want to do that same thing for fluency with polynomial operations, and I want to use visual patterns to do it."

Then I get home, check Feedly, and see that Michael posted a big essay on visual patterns and how kids think about them.

So I got busy reading. And it's great! I am worried that people won't read it because it's a PDF instead of a blog post. So consider this my heartfelt recommendation - Read Michael's essay. 

Below are some unconnected thoughts, critiques, and comments based on his essay.  I wrote them originally as a comment, so they are written to Michael.

1) On pages 2 and 3, you have a wonderful juxtaposition of a visual pattern and the number pattern 28, 14, 24. That was a big moment for me. I think i had a student all last year who I never got to understand patterns becasue she didn’t see how figure 3 grew from figure 2. She was also incredibly uncommunicative and disengaged, but thinking back, this may have been the source of her struggle.

What do you think about creating Figure 1, 2, and 3 using actual blocks on a document camera or something? It might help kids see how one shape builds from the previous one. I wonder what the downside of that is - framing all visual patterns as an extension from the previous figure. Maybe it limits kids’ ability to see the pattern in ways that don’t stem from the way it grows from the previous figure.

2) I have one kid who loves to find the common difference, multiply if by 40, and then add in Figure 3 to get figure 43. Where would you categorize that strategy?

3) "Relational thinking is great, but it’s not broadly useful” - Here is my first real disagreement. I think it's broadly useful in comprehending each function family, and therefore can be used in all sorts of useful situations. If I give students the sequence of numbers 3, 10, 29, 66, it's going to be hard for most kids to write a function for that sequence. But if I show them a nxnxn cube with 2 blocks stacked on top, many more kids could write a function. They wouldn't have to find the first, second, and third difference to determine that the function is cubic. They would see the cubic nature of the function from the cube.

To me, relational thinking is what connects linear, quadratic, and cubic functions to 1, 2, and 3-dimensional objects. It helps me see exponential growth such as  as a fractal in its 4th iteration, such as the picture below.

Having written two paragraphs about relational thinking, I am now wondering whether I have misinterpreted your definition of it, or whether the definition is still sort of inchoate and standing in for anything that isn't recursive or purely functional. 

4) “If kids stop seeing things recursively the project would collapse” Why? And do you think kids lose their recursive tools? Certainly not with linear growth. With quadratic growth, I can see it. But isn't recursive thinking less useful with nonlinear growth? Or, it's still useful but can be easily found from a table of values after the fact. I recognize that the slope of the function is represented by the first difference, etc. I wonder whether it's best to loop back to recursion once you're in formal-function-table-of-values land, rather than within a visual representation. Hmm.

5) Ooh, one big category of student thinking is improper applied proportionality. Finding figure 5 and multiply by 10 to get figure 50. How do we deal with that? It comes up a ton for my students.

6) I never thought of giving kids the rule for Fig n and asking them to draw a pattern. Seems fun, and the sort of thing you could post in the hallway to impress administrators. There could be a lot of cool colorful visual representations of 3n+5

Anyway, check out Michael's essay. It's very good, even if it's an unfinished draft.

Second (Grader) Impressions of DreamBox

Because I am a tiny bit obsessed, I commandeered my colleague’s computer and 2nd grade daughter, E, and let her play DreamBox for about 45 minutes.

Once again, I am very impressed by the depth and breadth of the activities available. For example, there are quick image activities just like for Pre-K, but instead of five circles, there are multiples of ten beads shown on a 100-bead rekenrek. E wanted to count by fives, but kept running out of time. After a couple of missed questions she switched to a count-by-tens strategy and blew through the lesson. It was really fun to watch a kid come up with a more efficient strategy, simply based on a well-designed activity. 

I had shown my colleague, Meredith, the videos of J, so she asked me to start filming E. I got a fantastic 90 second clip of her next activity.

The goal of this game is to make the same number a different way. In this case you were supposed to use the entire top row and as many additional beads from the bottom row. E has been getting through the lesson pretty easily when this happens:

Ok, so first she shows her awesome strategy for adding 8, which is to add 10 and count back by 2. You can hear her say “two less than 18” before she counts down to 16.

E used this strategy whenever the second addend was 7, 8, or 9. I have no idea what the standards look like for addition strategies, so I don’t know if this is explicitly taught, or something E came up with on her own. Either way, I loved the strategy.

So she uses it correctly on this problem, but when she goes to build her answer, that 17 that she said out loud gets stuck in her head and she builds the wrong answer. At this point, Meredith starts waving at me to stop recording and I swat her hand away because I AM CAPTURING AWESOMENESS. 

DreamBox says “Something is not quite right” but doesn’t specify the exact problem. E has to look back at her work and find her error, which she does. No adult needed to intervene in order for E to catch her error.

Let’s be clear: E knows how to count to 16 and build it in a different way. But she isn’t fluent yet. This is the sort of mistake that happens a lot when a child is becoming fluent with math. And it’s a vital part of the process. Notice how much more quickly she added 8+8 the second time. She is deepening these grooves in her mind as she practices problems that are within her comfort zone but not easy for her.

Then comes the second bit of awesomeness: Open number sentences! She has to represent what she did in an equation. I love this equation because it has a full expression on either side of the equals sign. So already, in second grade, E is learning that the equals sign represents equivalence, not “where the answer goes.” DreamBox even gives her the option of placing 16 in her answer, but she knows by this point in the game that the first number needs to represent the top row and the second number needs to represent the bottom row. Beautiful.

So E does some more activities, like one where she has to add two numbers, except one is expressed as a numeral and the other as a series of dots. Cool stuff.

Then we get to this activity, where I captured yet another AWESOME bit of student thinking.

E has to build her 100-bead rekenrek to match the one in the top left corner. She then has to find the numeral that matches the two-digit number she built.

E starts by counting rows, but she uses her count-by-fives strategy that I saw her use in an earlier activity. Except now she’s counting entire rows by fives. She realizes her mistake partway through and corrects herself, going back to count by tens.

Then she makes that exact same mistake five more times in a row. On every subsequent problem, she counts rows by fives before switching to counting by tens. On the fourth problem, she only catches her mistake when she sees that 25 isn’t available to click. Her mom, Meredith, is losing her mind in the background but doesn’t say anything because she knows that if you want your kid to learn sometimes you just have to shut up and let them make mistakes.

I thought, at the time, that she got the strategy right on the last problem, but watching the video I see that she never fully corrected this mistake. But if I were E’s dad or teacher, I wouldn’t mind one bit.

As I mentioned at the top, E just started using counting-by-tens as a strategy on a previous activity. It’s a new strategy, and one she’s less comfortable with than her count-by-fives strategy. So she’s trying to become fluent with her new idea but her old one keeps getting in the way. She’s regressing a bit in her counting as she tries to upgrade her strategy. Which is exactly what she should be doing. 

Every time I try something new in my classroom, I become a somewhat worse teacher in the short term. When a golfer tries to fix her swing, she gets worse at golf while she acclimates to the new motion. I have no doubt that E will become fluent at counting by tens. But she’s probably going to keep making mistakes for a while. And that’s fine.

I am so glad I caught that moment because it’s a great reminder to me as a teacher that students don’t automatically incorporate new information or correct their own misconceptions. Even if they can catch themselves, they are still prone to error as they build fluency. But as long as I can give them the time and space needed to become fluent. they will be fine.

After this afternoon of watching E play, I am even more convinced than I was last weekend of the value of this program. E is talking the way I want my students to talk and thinking the way I want my students to think. Not only that, after 45 minutes she turned to her mom and said "Can we play this some more when we get home?"

When my trial runs out, I am signing our family up. 

First Impressions of DreamBox

A little while ago, Tracy Zager wrote a fantastic post about the criteria she uses to evaluate math games. Within it, she recommended a program called DreamBox. It looked promising, so I installed it on my school iPad and signed up for a two week trial.

My son J and I just spent an hour playing with the games today, and I am very impressed. This is exactly the sort of math program that I have been waiting for years to see. 

A Ten Frame

I am so sick and tired of games that only care about “math facts.” I want a program that deals with math patterns, math representations, and math concepts. DreamBox, at least in the early levels, is full of the sort of math representations that I see in the elementary classrooms I envy. J was working with ten frames and rekenreks, which are fun to play with even digitally. He wanted to play each tutorial twice just because he liked sliding the rekenrek beads back and forth or changing the composition of the ten frame. 

A Rekenrek

There are even activities for the youngest learners, those who don’t even have a firm grasp on their numbers from 1-10. There is an early game, “More or Less,” where the game displays two quantities of dots and asks the student either “Which is more?” or “Which is less?” This game was great for J because he didn’t know the word “less.” I said it meant not as many which worked well for him until he got to a 1 vs. 2 problem and said “They’re both less!” I have learned a lot about talking math with my kids from Christopher Danielson, so I was elated to hear him say that. It started a fun little conversation about less and more.

Below, I have put a couple of 2-3 minute recordings of J playing through some other games. I have decided to write-up a detailed commentary of the math that I think is going on in each video. So consider this your chance to jump ship.

Check out DreamBox! I have no connection to this company other than I think they made a cool app. It’s got a free trial for 2 weeks and then costs $13 a month. That’s a pretty steep price. I’m still not sure if I’m going to pay it. We’ll see how the next 12 days go...

A DreamBox screenshot

Ok, now down here let’s get reeeeeel mathy. Remember, I am not an expert in this stuff. I am a middle school math teacher, but I have a hobbyist’s enthusiasm for early childhood math. So what I write is not a firm account of what happened in the video. It’s what I think is going on, and what I notice about that. So in the comments, please add your thoughts!

 Video 1

In this game, J has to replicate the top rekenrek using a double rekenrek (these are the abacuses with the red and white beads. I just looked up the name today, so don’t feel bad. I still don’t know how to pronounce it).

The thing is, he must use beads from both the top and bottom rows. I think the purpose of this activity is to get kids to move from a visual understanding of matching towards the idea of equivalence; that is to say, the idea that 2 and 3 are equivalent to 5. So that’s what J is working on.

So on the first problem, which I missed, J immediately yelled “2!” when he saw the two red beads. That means J can recognize the quantity 2 without counting the beads. This is known as “subitizing” and it’s a huge early math skill. It depends on the quantity and the arrangement of the objects. Look at the two pictures below. One you can look at and immediately know how many dots there are. The other, you can’t. It’s because the 5 dots are a smaller number and the dots are arranged in a very familiar pattern. The other one you actually have to count because there are more dots and they are scattered across the page.  

J can subitize 1, 2, 3, and sometimes 4. At this point, he hasn’t had to count any of the beads individually so far. So when he sees an amount he doesn’t recognize, he is in uncharted territory and asks me whether he can count. He counts up to 5, moves 4 across, asks me for help which I refuse to give, and then moves the fifth bead across. J then recounts his beads, gets 5, and celebrates by swinging the iPad around. 

Notice that he hasn’t touched the green button yet. The program hasn’t told J that he is right. J already knows for himself that he is right. That is what a conceptual understanding looks like.

Things I did that I hate:

“Now make 3 in a different way” - I did this because I already know (or think) that J can subitize 3, and I wanted him to focus on the mechanics of the game. But I should have said “Now make the next one in a different way.”  Let him do the work. No shortcuts, kid.

“Ooh, this is a hard one, J.” - This is such a tough habit to break. I’ve been a teacher for 6 years, and I’ve been trying to break this habit for 5.9 years. 

It’s so understandable. You want to build up kids’ confidence, so you see a challenging-but-solvable problem and you say “ooh, this one’s hard!” so that once they get it right, they feel smart. But the thing is, how do I know whether this problem is hard? J is going to try the problem in a second anyway. He can decide on his own whether the problem is hard or not. And if you ask him his opinion afterward, he might even tell you why.

But if I start the problem by saying “this one’s hard,” then I’ve already created an artificial sense of difficulty that colors his perception of the problem before he even starts to solve it. This is especially harmful when a child struggles to understand a problem that you’ve called “easy.” Every level of teacher does this, and it always hurts the kids. We start with easy and by middle school we say obvious and in college we say intuitive, but it all means the same thing. Which is “you better get this right pretty quickly or you’re dumb."

It’s better just to let the problem and J decide together whether or not it’s hard.

Things I did that I like:

“I don’t know. Try it.” - This is my standard answer when he asks for confirmation. J is more than 50% confident in his answer, but he’s looking to me to tell him he;s right. I don’t want to do that. I want him to get used to finding possible answers, thinking it through, and testing the ones he feels fairly confident will work. I want him to get comfortable with being uncomfortable.

All the parts where I wasn’t talking - Shut up and get out of the way, Dad. That silence is the sound of someone’s brain growing. Don’t interrupt it.

Video 2

This video was from the next game we played. In this game, you are supposed to make your ten frame match the given frame exactly and then click on the numeral that matches the frame. J is pretty familiar with the numerals from 1-10, but this is one of several games that helps to strengthen that connection. The numbers are laid out in order so you can count up to the appropriate numeral if you don’t recognize it by sight. 

Since we had just been working on equivalence, I think J is just trying to match the quantity. I think he didn’t realize that his ten frame had to look exactly the same as the frame above it. So that’s what I’m explaining to him at the beginning.  

First thing: J can subitize 10 in a ten frame! That is news to me, and very impressive considering he just saw ten frames for the first time today. Unless they’re using them in his pre-school class already, which I doubt, this means he has learned a new idea from DreamBox already. Also, he knows that moving the 10 dots at once is more efficient than moving them 1-by-1.

J decides the next problem, nine, is a tough one without me even saying anything! See, he does have his own internal sense of difficulty. So he counts the nine dots, sings a little adorable Nine Song, and starts to build his nine in a very efficient manner, by starting with the 5-dot piece and then getting the 3-dot piece. Then he concludes he’s done. I try to ask a question without giving away that he’s made a mistake. 

So he counts his frame, gets eight, goes back and counts the original frame, has a little I’m-a-three-year-old moment, and just gets a bit discombobulated. So I ask him to put down the number he thinks it is, and he picks 9. I find this interesting since he didn’t get nine for either of his most recent counts. But the correct answer was still in his brain somewhere. If you go back and look, just after the Nine Song is a moment when his finger hovers over the numerals. I think he was about to pick 9 then, so he made that connection before he even started building the ten frame.

I am in love with this second video. For one thing. I did a much better job of getting out of J’s way and letting him reason through the problem. 

Secondly, I love that J made mistakes in this video. He sees two similar ten frames as being the same, and he doesn’t properly count the original frame on his second attempt. The concept of touching one object per number when counting, which is called one-to-one correspondence, is something that J is decent at but still messes up occasionally. When he gets off track in counting, he starts singing the numbers in sequence but is no longer connecting them to the dots. 

AND THAT IS OKAY. He is a three year old and working on a new skill. I don’t need to stop him and correct him every time I see him make that sort of mistake. The program will catch J’s mistake and over time he will become a more precise counter. I love mistakes. It means that kids are working at the edge of their abilities. And I don’t always correct J, any more than I correct every little grammar mistake he makes. J used “he” and “she” interchangeably for almost a year before he realized that they connoted gender. I never corrected him for months and months. I just used the pronouns correctly in my own speech and figured he’d figure it out when he had a firm grasp of “boys” and “girls” as an underlying concept. And you know what? Almost a year later, he was ready. 

What I care about is that the program found his mistake and asked him to try again. This is one of Tracy’s 3 big criteria for math games, and it’s spot on.

So right after I shut off the video, J picked up a 5-dot piece and then filled in the second row one-by-one until he got to nine. Then he said “It’s easier to do ones” and on the next one, which was a seven, he filled in all seven dots by ones. He shifted to a less efficient strategy that was within his comfort zone. Again, I am fine with this. Over time, he will shift back to using the larger pieces once he finds single-dot pieces too time-consuming to use. I’m not going to force it.

So J, like every other child in the entire world, isn’t a perfectly elegant problem solver yet. But he’s a little bit better than he was at the beginning of the day. Credit where due: DreamBox has put together a very promising app. I am really looking forward to the next 12 days. 

Permit Me to Brag

My Algebra 1 students surprised me. I typically hate grading final exams, but as I was grading my students' exams I noticed something that got me legitimately excited! I was actually, enjoying the process of seeing each new student's work.

Specifically, I wanted to see how they solved problem #16.

When I wrote the question, I had anticipated that students would use a couple of different strategies. What I didn't know was that my 25 students would use a combined seven correct solution strategies to solve this problem.

Here they are, starting with the ones I anticipated.

Eight students started at (7,4) and used the slope to fill out a table or list of points until they reached the point (13,0).

Six students substituted in 13 for x and solved for y.

Four students substituted in 13 for x and 1 for y and got an impossible solution.

Now we get to my favorites:

One student, W, started at (13,1) and worked backwards up to (7,5) to determine that (7,4) was not on the same line.

One student, K, used a coordinate plane from another page to graph the line and then wrote his justification down. He erased the points, but I found them, very faintly, on the next page. 

Clever girl... (Well, he's a boy, but still)

One student, E, found the y-intercept of (0, 8 2/3) and then made a table of values from that point until he was satisfied that the line never intersected (13,1).

And my absolute favorite, the most elegant of all the solutions: One student, M, found the slope between (7,4) and (13,1) and saw that it was different from the slope of the line. QED.

Way to go, M!

(Side note: Three students got the problem wrong. Two of them substituted the y coordinate in for x, and the last student mixed up her slope and used -3/2 instead of -2/3)

I want to be clear as to why I am so thrilled. It is not because Problem 16 is some deep, challenging math problem. It's pretty straightforward and simply requires an understanding of point-slope form. It's easy to teach kids a procedure to solve it.

But I'm proud because I never explicitly taught a procedure for solving this problem. Instead, I gave a similar problem to my students in class, let them work on it in groups, and shared some of my students' various solution strategies. There was never a moment where I said "Ok, from now on always make sure to substitute this point into this equation to determine whether that point lies on the line described by the equation."

To be honest, I really didn't know at the time whether I was doing the right thing. Sure, everyone got the problem right in class (in groups, with support), but without a single procedure or mnemonic, would they be able to do it again weeks or months later?

The answer, I'm comfortable saying, is yes. My students retained the concept behind the problem. Different students chose strategies that worked best for them. Sure, E did a bunch of extra work when he found the y-intercept and made a table of values. But he used the method that made sense to him. He stopped trying to recall a set of steps and instead said "What can I find out from this information, and how can I use it to solve the problem?" M used the same approach and came up with a strategy that I had never seen until I was grading her exam. Her strategy is so elegant I can't believe I never got the chance to show it off in class!

To be honest, that's why I wrote this post. I want to brag on M. But really, I want to brag on all my students. Other kids came up with cool strategies on other problems. Kids surprised me on a final exam. My students are acting like mathematicians, y'all. They're using their toolkit of math ideas to solve problems flexibly. I couldn't be happier.


 

Ok, if you've followed me down here, you can read this part. I'm still secretly scared that I'm doing it wrong. Every time I get all puffed up and start to crow, a little voice in my head starts talking about how pride comes before the fall. Because after all, E doesn't have an efficient way to solve this problem. For that matter, neither does K. So did I really teach those kids how to solve this type of problem? Duct tape can hold up a light fixture if you use enough, but that doesn't mean it's up to code.

What do you guys think? Should I be proud? How can I keep improving so I reach those students who found creative-but-inelegant solutions? What about the three who missed the question - what do I do about them?

One Week in Mr. Haines's Math Class - Friday

Friday

Warm-up:

I ask students to look at their worksheet from yesterday and pick one acute triangle, one right triangle, and one obtuse triangle. I then call on 3 kids at random to share their side lengths.

Activity:

I've gone through a great deal of discovery work yesterday, so it's time for a little guided instruction. I give a small lecture about the Greeks, who were faced with a similar problem. Except the Greeks noticed something pretty cool that happens when you square the sides...

I square the sides for each triangle and ask students to look for patterns for 2 minutes. In each class, someone noticed that the right triangle's short sides added up to the long side when all sides were squared.

So we use that as a springboard to analyze acute and obtuse triangles. Pretty quickly, we see that acute triangles' short sides add to more than the longest side, whereas obtuse triangles' short sides add to less than the long side. (I keep saying, over and over, "after you square all the sides" like a broken record because I am terrified of students forgetting that step.)

So now we have a hypothesis. How do we test it? Three new triangles! I get three new triangles from my students and try out our hypothesis, which seems to work!

Time for notes in our $1 Textbooks. We write down the Pythagorean Theorem, a diagram with the legs and hypotenuse defined and labeled, and the rule for classifying triangles using only their sides.

Finally, I give students a final triangle worksheet. In this worksheet, they are given the sides but not provided a ruler. They must use this new classification tool to classify the triangles.

If we have time, I've embedded an extension task in this worksheet. In the first problem, we had sides of 6, 8, and 11, which resulted in an obtuse triangle. The second problem, 6, 8, 8, resulted in an acute triangle. What would we need the hypotenuse to be to make a right triangle?

Homework

None

Monday's Goal:

Continue using the Pythagorean Theorem, now finding the missing sides of various triangles.
 

Resources:

Classification Worksheet

One Week in Mr. Haines's Math Class - Thursday

Thursday

Warm-up:

None - we have a lot to do today!

Activity:

I hand out two worksheets - a set of triangles that I have drawn and a worksheet where students will collect their work for the day. I also give out a ruler to each student. The instructions are simple: Using the centimeter side of the ruler, measure all three sides of each triangle. Then classify each triangle by its sides and by its angles.

For the file, check the link above or the Resources section below,

I had to hand-draw these triangles using a compass and ruler to ensure that the measurements were precise, but that's fine - I love constructing geometric figures. (In fact, I think kids should spend WAAAAY more time in geometry constructing figures of their own, but that's a side issue.)

This section of the class whips by pretty quickly, and I was able to help out any students who were struggling with using a ruler. They can all use rulers, but only if I really, truly force them.

On the back of their classification worksheet, I list a bunch of triangles by their sides and ask students to classify these triangles by their sides and angles. Pretty quickly, they realize that all the triangles are scalene, but how can you tell whether they are acute, right or obtuse? Mr. Haines? Mr. Haines? Can you come here?

At this point, I am walking from table to table distributing scratch paper and encouraging students to try to draw each triangle. There is a LOT of trial and error as students draw, then redraw, then redraw their 8, 9, 10 triangles or their 2, 8, 9 triangles. I don't worry too much about this. After all, I am giving the students a headache so they appreciate the aspirin.

Also, these are some really clever students I'm teaching! In two of my three classes, I had a student come up with the following strategy, which I will paraphrase:

"I pretend the triangle is a right triangle. So I draw the short side and the medium side with a right angle, and I try to connect them with the third side. If the third side reaches perfectly, it's a right triangle. If it's too short, the triangle is acute because the short side has to bend down to reach it. If the long side is too long, the triangle is obtuse."

Pretty cool, right?

By this point, we are edging right up against the bell, so I bring the students together, run through a quick check of their classifications, and then ask them why it took them so long. Lots of grumbling about erasing and redrawing. I mimic every announcer from every infomercial eve: "There's got to be a better way!"

The bell rings. Tomorrow, we meet Pythagoras.

Homework

None

Tomorrow's Goal:

Introduce the Pythagorean Theorem and use it to classify triangles.

Resources:

Triangle Worksheet (I tried very hard to get the scale of the triangles to remain after scanning and converting to a PDF. Hopefully this works for you, but it may depend on your printer. Or you could construct your own set of triangles!) 

Classification Worksheet 

One Week in Mr. Haines's Math Class - Wednesday

Before we get into today's lesson, which was my favorite lesson all week, can I rant about something for a minute?

Why in the world would someone try to teach about square roots without talking about squares? I'm not referring to "squares" as in raising a number to the second power. I'm talking about "squares" as in those pointy shapes with all the sides that match each other.

You will notice that I didn't even introduce the notation or the term "square root" until my lesson on Tuesday. This was intentional. I don't want my students to get hung up on this new vocab term or this symbol that kind of looks like a long division sign. No, I just want them thinking about how to find the length of one side of a square that has an area of 73.

So already, on day 1 of this unit, I have students who are accurately estimating square roots. They just don't know that they're doing it yet. They think they're finding the missing side of a square. Once they know that concept and build a strategy to solve that sort of problem, it's not a major shift to tell students "Ok, that thing you've been doing, where you un-square a number? That's called a square root. And it looks like this check-mark-with-a-bar-next-to-it symbol you've noticed on your calculator."

Conversely, if you start this unit by projecting a slide entitled "Square Roots" and introduce all the formal vocab and symbols from the start, then students don't have anything to ground their understanding of the operation. You are asking students to use a new symbol to enact a new operation that they've never tried before. And forget about asking them to find the missing side of a square - that would be a seemingly-impossible task to those students.

Introduce the challenge first. Once students understand the challenge, then provide the notation and the vocabulary. Don't dump them both into students' laps at the same time.

Ok, rant over.

Warm-Up:

None. We are diving into the main part of the lesson, something I have been dying to try since I saw Andrew Stadel post about it back in August.

Activity: Movable Number Line

My number line is huuuuge. Great for class, hard to capture in a picture.

Ok, so this might have been the most awesome thing I've done all year. I made a loooooong number line out of a string that stretches almost all the way from my window to my door. I put the numbers 0 and 10 on either side of the number line.

I told kids that I would be showing the whole class a number and then calling on one person to place that number on the number line. Nobody else could talk, but we would take a poll after the student sat down:

• Thumbs Up: Perfect!
• Thumbs Sideways: Your answer is in the correct order but needs to slide either right or left
• Thumbs Down: Your answer is out of order

Then I held up the number 4. I chose 4 on purpose because it's incredibly familiar to students and yet a bit tricky to place. It's closer to 0 than to 10, but how much closer? Not to mention, with such a long number line, it's going to be hard to get the placement exactly right. I was expecting a lot of "Thumbs Sideways" on this first number, and I wasn't disappointed.

By the way, I use popsicle sticks with names, often called equity sticks, to choose my participants in class for this activity. I know that some teachers feel that equity sticks cause students anxiety, but I think they are worth it for this sort of activity. First of all, they strongly improve engagement. Everyone knows that they could be called up to place the next number, so they are paying attention to each number I present. Secondly, this activity does not have a clear right-or-wrong answer. In fact, I usually end up polling the class and sliding each answer ever-so-slightly in one direction or the other. Since every answer gets improved or amended, the pressure to be exactly right is lowered. Everyone is just making their best guess.

But back to the game. The first student has just placed the number 4, and we have to decide - is it perfect, or should it slide right or left? In my first class I had a student place 4 verrry close to 0. This is a great opportunity to ask students to critique the reasoning of others in a respectful way. I had lots of great comments from students, such as "If 4 was that close to 0, you wouldn't have room for 1, 2, and 3, and you'd have way too much room for the numbers bigger than 4." As the lesson went on, the justifications became more precise.

My next choice, root(49), I chose because 7 is exactly halfway between 4 and 10, and I want to see fi my students will pick up on that.

My third choice, root(20), is where the real fun starts. Now students have to use yesterday's skill of estimating square roots without any benchmarks. Where should root(20) go? Where is 5 on this number line? Where is 6? These are all questions that my students are silently asking themselves as I hold up the card with root(20) on it. At least, it sure seems that way. My students are rapt. They can't wait to find out if their popsicle stick will get pulled.

From here, my sequence was 6, root(93), root(40), root 4, root(14), root(-4). I'm sure I could have sequenced them better, but I'm not sure how. How would you sequence this activity? Let me know in the comments.

Are these placed appropriately? If you could slide one card, which one would you slide, and where?

Are these placed appropriately? If you could slide one card, which one would you slide, and where?

Anyway, I like this lesson for a few reasons:

• Student engagement was through the roof. I felt like everyone was with me in a way that almost never happens. Kids were having fun! I even had a group of students ask me in study hall the next day if they could play "the number line game" again
   
• Because the number line is huge, almost nobody placed their card in exactly the right spot on the first try. We always had to shift someone's card a little to the right or the left. Conversely, almost nobody put their card in the wrong order. So all the students were participating in an activity where nobody got an answer totally wrong, but nobody got an answer totally right. The pressure that students feel when coming to the front of the class was lessened in this case. Sometimes, students had a legitimate difference of opinion and we had to agree to disagree since this activity has an inherent amount of imprecision. But that's great! I'd rather my students be disagreeing and debating as long as they back up their ideas with some evidence
   
• By placing radicals on a number line, students are beginning to interact with radicals as objects that have a specific value. It's not just a problem to be solved. Root(14) is a number that is somewhere between 3 and 4. That approach to radicals will be useful in Algebra 1.
   
• I threw root(-4) in as a challenge because I thought it would spark a great debate. I also wanted to add a little bit of new information into the day's material. Yes, it's a trick question. Maybe it will feel more memorable to students because they spent 3 minutes arguing over the location of root(-4) before I admitted that there is no place for this answer on the number line. At least, not this number line.

This took about 30 minutes, which sounds crazy, but it was my first time trying a number line, and we had great discussions between every number.

Here’s a good extension. Start with numbers other than 0 and 10. Here I’ve started with 2 and 8. Where would you place root(36)? Root(90)? Root(3)?

Once we had placed all the numbers on the number line, I pulled more popsicle sticks and got students to sort the numbers on the number line into the categories "Rational" and "Irrational." This is my attempt to cement the idea of irrational numbers within our existing activity. Kids could sort the numbers perfectly, but I still don't know if they truly understand what irrational numbers are, and how they actually differ from rational numbers. Something to think about before next year.

Lastly, I got students to create a foldable for their $1 Textbooks to help them classify triangles by their sides and angles. This is vocab that they should know, but it's going to be vital for tomorrow's activity, so it's worth the time investment to make a good foldable.

Note to self: draw triangles in the foldable so kids don't draw their own "obtuse" triangles that are clearly acute.

Homework

IXL Activity on estimating square roots. I am lagging in my implementation of lagging homework. I'll get better at this.

Tomorrow's Goal

Classify triangles by their sides and angles, maybe even discover the Pythagorean Theorem?