I finally got a pattern machine in front of my four-year-old son J, and it has been love at first sight. He loves the bright colors, the satisfying cli-click that each button makes, and the endless possibilities for designs and patterns. So far he has made a tree with a sun and a moon, a row of blaster guns, and a house.
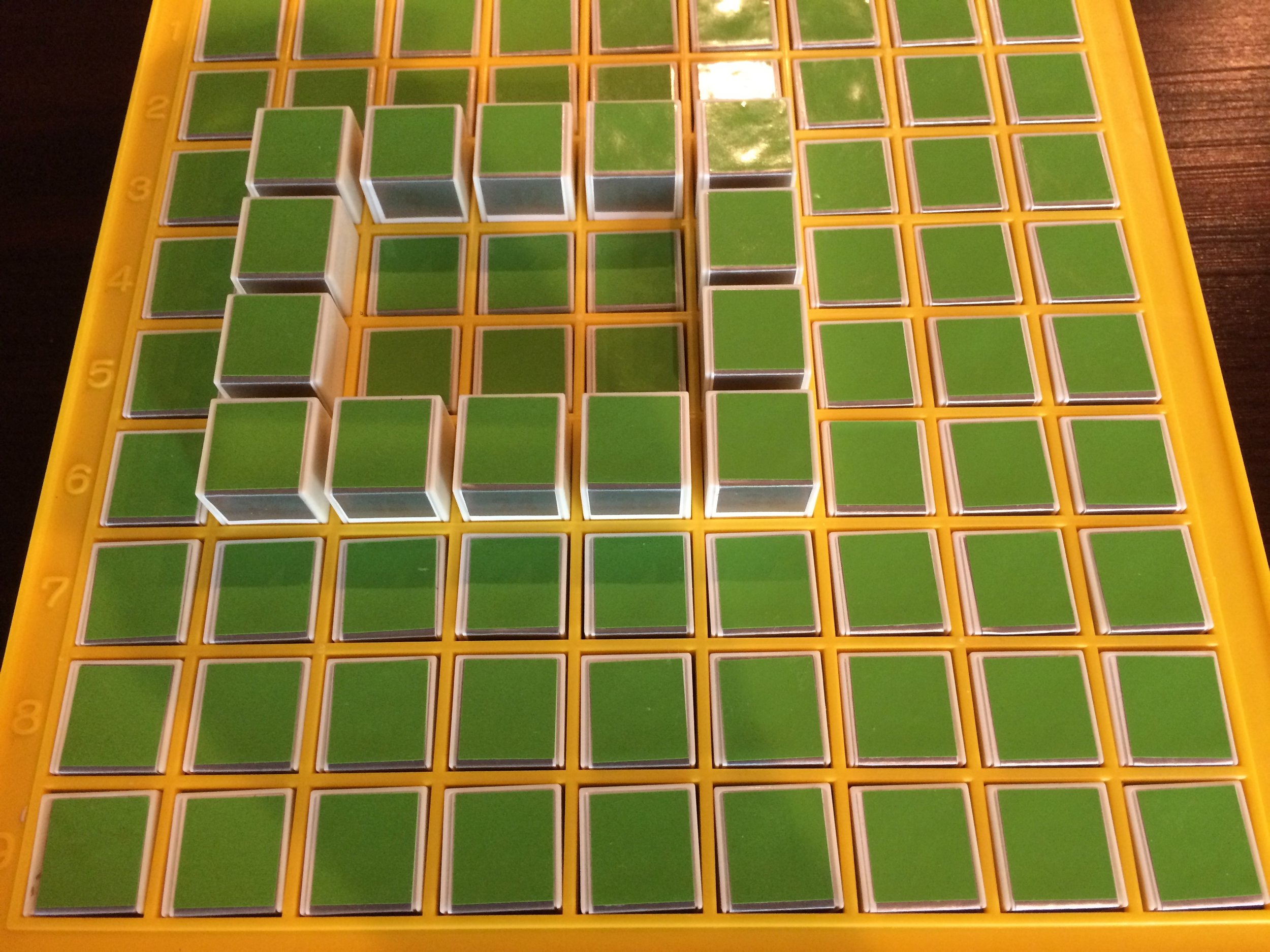
On Saturday, J made this shape. "Look Daddy, a square!"
I saw an opportunity to firm up his definition of a square. "Count the sides."
J counted the bottom side and got five, but then had trouble deciding where to start counting along the right side. After all, he had already counted the bottom right square as part of the bottom. Was it part of the side as well?
This concept of when and how to count, like the concept of halfway, is something I have seen as often in my middle school class as I've seen from my son and his friends. Typically my middle schoolers struggle to count grid lines to find slope or translate a triangle four spaces to the right. Should the count boxes, or the edges of the boxes? How do you use discrete objects to measure continuous distances?
These measurement ideas are certainly something I want to read more about, but I'll have to save that for later. What I did instead was tell J to start counting at the corner. He did so and got a length of four. I told him "Squares have four sides that are all the same. So this rectangle is not a square. Can you make a square?"
This was his second draft. Again, I said "Count the sides" and noticed that he still hesitated before double-counting the corner square. He got sides of six and four. I said "Still not a square." Then he made the one below. He sat back triumphantly and said "This one is definitely a rectangle."
Once again I asked "Can you make a square?" He pushed all the buttons down, then made a C shape that was four blocks high and three blocks wide, and finally popped up the four boxes on the right to make a square!
I didn't want to give away the answer, so just like all the other times I said "Count the sides." By this point, J felt comfortable counting his sides, so he counted four along the bottom and four along the right side. I almost said "Congrats!" But then realized that he wasn't done. He continued counting along the top and left sides, confirming that each side did indeed have a length of four.
Of course! It felt so obvious to me that counting the base and height was sufficient to prove the shape's squareness, but why would J know that? He needed this moment after he constructed the shape to confirm its attributes. Over time, he may notice that the bottom and top sides always have the same measure, but for now he needs to play with these shapes more and discover these properties on his own.
So thank goodness for the pattern machine, which gives J a developmentally appropriate way to construct and examine these shapes! J still has trouble drawing straight lines and connecting endpoints, so of course he's limited in the shapes he can make by hand. He benefits greatly from the discrete structure of the pattern machine, which ensures straight lines and right angles.
I also realized that he and his classmates had been exploring other shapes at pre-school, using pretzels to form triangles and other shapes. Check out these pictures from the album that my son's awesome preschool (Yay Chai Tots!) sends out every week. Once again, J and his classmates are using tools appropriate to their age to build and analyze shapes, instead of simply looking at them on a page.
I mention all this because my 8th grade students struggle with geometric concepts more than any other topic in math. When teaching square roots, I like to start by finding the area of squares, which is definitely not an 8th grade topic. Yet I still have to provide enormous scaffolds to ensure that my students know what area is and how to find the area of squares and rectangles.
I don't know why my students struggle so much with this topic, but I have a theory. I don't think they've spent enough time constructing and analyzing shapes of their own design.
Think about it: if you've never used a compass to draw a circle, why would you care about its radius? We don't care about the "radius" of a triangle or a rectangle or any other shape (at least, not in middle school). But if you actually try to draw a circle, the benefit of the radius becomes immediately clear. I know that I looked at circles for years and years without ever considering that they were the set of all points equidistant from some center. Then one day in 7th grade I used a compass and the lights came on.
The same tendency is true of the triangle inequality theorem. This theorem, if not experienced directly, seems bizarre to students. The sum of any two sides of a triangle must be greater than the third side? Ok, sure, Mr. Haines.
But if you give kids rulers and ask them to draw a triangle with sides of 3, 4, and 17 centimeters, they will quickly realize that the task is impossible. And then you can begin a conversation about which triangles are and are not possible. What if the longest side were 10? 8? Exactly 7 centimeters?
Mostly, I don't think students spend enough time actually constructing and analyzing shapes directly. Heck, most students rarely find the actual area and perimeter of shapes. Instead, they are given shapes with pre-labeled sides that might not even be accurately scaled!
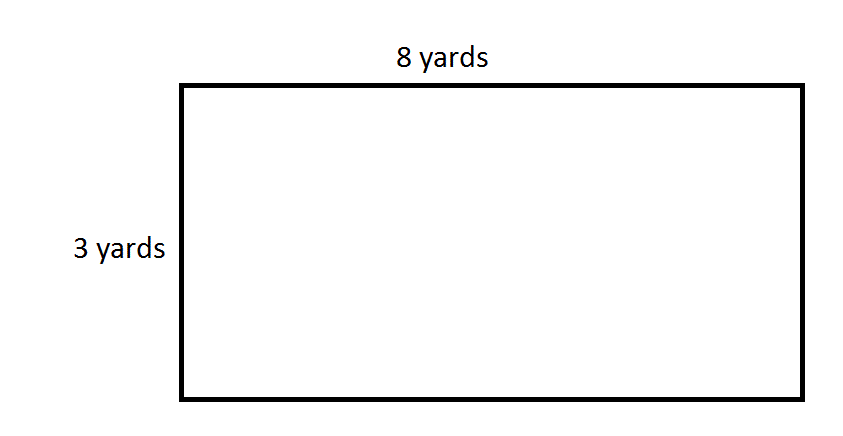
Look at this rectangle, which was the third Google Image result for the phrase "area of a rectangle." Does the base really look like it's almost three times as long as the height?
You might think that these questions are harmless, that they are a good way for students to practice finding area and perimeter. But I disagree. I think that these questions actively undermine our students' visual intuition. I think that as students solve problem after problem of impossibly-labeled shapes, they learn to ignore that spidey sense in the back of their minds that says that these numbers can't possibly be right. I think these problems make our students less capable of solving geometry problems, not more.
This blog really took a turn! I am wondering so many things right now. I wonder what percentage of geometric figures in major textbooks are labeled accurately. I wonder whether the rectangle above is the sort of error that a 3rd grader is more likely to notice than an 8th grader. I wonder how many students who could find the area of that 3x8 rectangle could find its actual area if I printed it out, whited out the "measurements," and handed them a ruler.
Most of all, I wonder who else has been thinking about this stuff. Let me know in the comments whom I should be reading.